Month: September 2023

Differences Between Enthalpy And Entropy

Class 11

Posted by : konetwon / On : September 15, 2023

Differences Between Enthalpy And Entropy: In the realm of thermodynamics, two fundamental concepts play pivotal roles in understanding and describing the behavior of matter and energy: enthalpy and entropy.

These concepts are often used in chemistry, physics, and engineering to analyze and predict the outcomes of various processes. While both enthalpy and entropy are thermodynamic properties, they have distinct definitions and applications.

In this article, we will explore the key differences between enthalpy and entropy and how they contribute to our understanding of the physical world.

Differences Between Enthalpy And Entropy

Enthalpy: The Measure of Heat Content

Enthalpy (H) is a thermodynamic property that represents the total heat content of a system at constant pressure. It includes the internal energy of a system and the energy required to maintain the constant pressure during various processes, such as chemical reactions. Enthalpy is often used to describe heat transfer in chemical reactions and phase changes.

Key characteristics of enthalpy:

Heat Exchange: Enthalpy accounts for the heat exchanged between a system and its surroundings. In exothermic reactions, the enthalpy change is negative, indicating a release of heat energy. In endothermic reactions, the enthalpy change is positive, signifying an absorption of heat energy.
Constant Pressure: Enthalpy is particularly useful at constant pressure because it directly relates to the heat exchanged in open systems, like those commonly encountered in chemistry laboratories.
State Function: Enthalpy is a state function, meaning it depends solely on the initial and final states of a system, not on the path taken to reach those states.

Entropy: The Measure of Disorder

Entropy (S) is another thermodynamic property, but it deals with the degree of disorder or randomness within a system. It is a measure of the system’s tendency to move from a state of order to a state of disorder. Entropy is used to explain why natural processes tend to favor disorder over order.

Key characteristics of entropy:

Disorder: Entropy is a measure of the randomness or disorder within a system. Higher entropy values indicate a more disordered state, while lower values indicate a more ordered state.
Second Law of Thermodynamics: The second law states that in any energy transfer or transformation, the total entropy of an isolated system always increases over time. This principle underlines the irreversibility of natural processes.
Microscopic Level: Entropy is a microscopic property that relates to the statistical distribution of particles and their energy in a system.

Comparison

Now, let’s summarize the key differences between enthalpy and entropy:

1. Definition:

Enthalpy (H): Measures the total heat content of a system at constant pressure.
Entropy (S): Measures the degree of disorder or randomness within a system.

2. Focus:

Enthalpy focuses on heat exchange during chemical reactions and phase changes.
Entropy focuses on the tendency of natural processes to move towards greater disorder.

3. Symbol:

Enthalpy is represented by H.
Entropy is represented by S.

4. Unit:

Enthalpy is typically measured in joules (J) or kilojoules (kJ).
Entropy is typically measured in joules per kelvin (J/K).

5. State Function:

Enthalpy is a state function.
Entropy is a state function.

Conclusion

Enthalpy and entropy are essential concepts in thermodynamics that provide valuable insights into the behavior of matter and energy in various systems. Enthalpy deals with heat content and exchange, particularly at constant pressure, while entropy quantifies the degree of disorder within a system and explains why natural processes tend towards greater randomness. Understanding the differences between these two properties is crucial for scientists and engineers working in fields where thermodynamics plays a significant role, as it enables them to make predictions and optimize processes more effectively.

Read More

Frequently Asked Questions (FAQs) Differences Between Enthalpy And Entropy

What is enthalpy, and how does it differ from entropy?

Enthalpy (H) is a thermodynamic property that represents the total heat content of a system at constant pressure, including the internal energy and energy required to maintain constant pressure during processes. Entropy (S), on the other hand, measures the degree of disorder or randomness within a system. Enthalpy deals with heat transfer, while entropy deals with the tendency of natural processes to become more disordered over time.

What are the main units of measurement for enthalpy and entropy?

Enthalpy is typically measured in joules (J) or kilojoules (kJ), whereas entropy is usually measured in joules per kelvin (J/K).

How do enthalpy and entropy relate to chemical reactions?

Enthalpy is crucial in describing the heat exchange that occurs during chemical reactions. Exothermic reactions release heat energy, leading to a decrease in enthalpy (ΔH < 0), while endothermic reactions absorb heat energy, resulting in an increase in enthalpy (ΔH > 0). Entropy, on the other hand, explains why chemical reactions tend to move towards states of greater disorder or randomness.

Are enthalpy and entropy related to each other?

Yes, enthalpy and entropy are related through the Gibbs free energy equation (ΔG = ΔH – TΔS), where ΔG is the change in Gibbs free energy, ΔH is the change in enthalpy, ΔS is the change in entropy, and T is the temperature in kelvin. This equation helps determine whether a process is spontaneous (ΔG < 0) or non-spontaneous (ΔG > 0).

Are enthalpy and entropy state functions?

Yes, both enthalpy and entropy are state functions. This means that their values depend only on the initial and final states of a system and are independent of the specific path taken to reach those states.

Difference Between Work And Energy

Class 10

Posted by : konetwon / On : September 14, 2023

Difference Between Work And Energy: In the realm of physics, the concepts of work and energy are fundamental and interconnected. Both play essential roles in describing and understanding the physical world.

However, they are distinct concepts with specific definitions and units of measurement. In this article, we will delve into the difference between works and energy, their definitions, units, and practical applications.

Difference Between Work And Energy

Work:

Definition: Works, in physics, is defined as the product of the force applied to an object and the distance over which the force is applied in the direction of the force. In mathematical terms, it is expressed as:

$cos (θ)$

Where:

$W$ represents work in joules (J).
$F$ represents force in newtons (N).
The variable ‘d’ symbolizes the distance, measured in meters (m), over which the force is exerted.
$θ$ represents the angle between the force vector and the direction of motion.

Units: Work is measured in joules (J), which are equivalent to one newton-meter (N·m).

Characteristics:

Work is a scalar quantity, meaning it has only magnitude and no direction.
Work can take on positive, negative, or zero values depending on whether the force is exerted in the same direction as the displacement, in the opposite direction, or when no work is performed, respectively.
Work is linked to the conversion or transfer of energy from one object to another or from one energy form to another.

Energy:

Definition: Energy is the capacity to do work. It is a scalar quantity and exists in various forms, including kinetic energy (energy of motion), potential energy (energy due to position or configuration), thermal energy (heat), chemical energy, and more. The total mechanical energy of an object is the sum of its kinetic and potential energies and remains constant in the absence of external forces.

Units: Energy is quantified in joules (J), which is the identical unit used for work.

Characteristics:

Energy is a scalar quantity, like work, and has no direction.
Energy can change from one form to another, but the total energy in a closed system remains constant (law of conservation of energy).
The various forms of energy are interconvertible. For instance, potential energy can be converted into kinetic energy, and vice versa.

Key Differences:

Definition: Works is the product of force and displacement in the direction of the force. Energy is the capacity to do work or the ability to cause changes in a system.
Units: Both work and energy are measured in joules (J).
Direction: Work is a scalar quantity devoid of direction, whereas certain forms of energy, such as kinetic energy, can be linked to a specific direction, such as the direction of motion.
Change: Work represents the transfer of energy from one system to another, while energy can change from one form to another within a system.

Practical Applications:

Work finds application in the calculation of the mechanical effort required for a wide array of tasks, encompassing activities such as lifting objects, propelling vehicles, and various other endeavors.
Energy is the basis for understanding the operation of engines, power generation, and the behavior of physical systems.

In summary, while works and energy are closely related concepts, works represents the specific action of transferring energy through the application of force over a distance, while energy is a more general concept that encompasses various forms and is the capacity to do work. Understanding these concepts is essential in physics, engineering, and everyday life for analyzing and solving a wide range of problems and scenarios.

Read More

Frequently Asked Questions (FAQs) Difference Between Work And Energy

1. What is the fundamental difference between work and energy?

Work encompasses the transfer of energy between objects or the conversion of energy from one form to another. Its precise calculation involves multiplying the applied force by the displacement that occurs in the direction of the force. In contrast, energy signifies the capacity to execute work or bring about changes within a system.

2. How are the units of work and energy related?

Both works and energy are quantified using the unit of joules (J), which is equivalent to one newton-meter (N·m).

3. Can energy have a negative value?

Energy is a scalar quantity and can be negative, positive, or zero depending on the context. For example, an object in a lower gravitational potential energy state may have negative potential energy relative to a reference point.

4. Is work a vector or scalar quantity?

Works is a scalar quantity because it has magnitude but no direction. It can be positive, negative, or zero.

5. How does energy conservation relate to work and energy?

Energy conservation is a fundamental principle stating that the total energy of an isolated system remains constant. Works done on or by the system can change the distribution of energy among its various forms, but the total energy remains conserved.

Difference Between Concave Convex Lens

Class 10

Posted by : konetwon / On : September 14, 2023

Difference Between Concave Convex Lens: Lenses are essential optical components that play a crucial role in various optical devices, including cameras, telescopes, microscopes, and eyeglasses.

Among the different types of lenses, concave and convex lenses are two primary categories, each with distinct properties and applications. In this article, we will delve into the key differences between concave and convex lenses, their shapes, characteristics, and applications.

Difference Between Concave Convex Lens

Concave Lens:

1. Shape and Structure:

A concave lens is thinner at the center and thicker at the edges. It curves inward, resembling a cave or a bowl.
The lens has two curved surfaces: one concave (curving inward) and one flat.

2. Light Refraction:

When parallel rays of light pass through a concave lens, they diverge or spread apart.
The point from which the diverging rays appear to originate is called the focal point (F), which is on the same side as the object.

3. Image Formation:

A concave lens forms virtual, upright, and diminished images for real objects placed beyond its focal point.
It can also create a virtual image for an object placed between the lens and its focal point.

4. Applications:

Concave lenses are commonly used in corrective eyeglasses to treat nearsightedness (myopia).
They are also used in devices like movie projectors to spread out the light from a small source and create a larger image.

Convex Lens:

1. Shape and Structure:

A convex lens is thicker at the center and thinner at the edges. It bulges outward, resembling a lentil or a magnifying glass.
The lens also has two curved surfaces: one convex (curving outward) and one flat.

2. Light Refraction:

When parallel rays of light pass through a convex lens, they converge or come together.
The point where the converging rays meet is called the focal point (F), which is on the opposite side of the object.

3. Image Formation:

A convex lens forms real, inverted, and magnified images for objects placed beyond its focal point.
It can also create virtual, upright, and magnified images for objects placed between the lens and its focal point.

4. Applications:

Convex lenses are widely used in eyeglasses to correct farsightedness (hyperopia) and presbyopia.
They are essential components in cameras, telescopes, and microscopes for focusing and magnifying distant or small objects.

Key Differences:

Shape: Concave lenses are thinner at the center and thicker at the edges, while convex lenses are thicker at the center and thinner at the edges.
Light Refraction: Concave lenses diverge incoming light rays, while convex lenses converge them.
Focal Point: In concave lenses, the focal point is on the same side as the object, while in convex lenses, it is on the opposite side.
Image Formation: Concave lenses produce virtual and diminished images for objects beyond the focal point, while convex lenses create real and magnified images for such objects.
Applications: Concave lenses are used for correcting nearsightedness and in devices like projectors. Convex lenses are used for correcting farsightedness and in optical instruments like cameras, telescopes, and microscopes.

In summary, concave and convex lenses have distinct shapes, behaviors, and applications in optics. Their ability to refract light in different ways makes them invaluable in a wide range of optical systems and corrective lenses, enhancing our ability to see and manipulate the world around us.

Read More

Frequently Asked Questions (FAQs) Difference Between Concave Convex Lens

1. What are concave and convex lenses primarily used for?

Concave lenses are often used in eyeglasses to correct nearsightedness, while convex lenses are used to correct farsightedness. Both types are used in various optical devices, including cameras, telescopes, and microscopes.

2. How do concave and convex lenses differ in their shape and curvature?

Concave lenses are thinner at the center and thicker at the edges, curving inward. Convex lenses are thicker at the center and thinner at the edges, bulging outward.

3. What happens to light when it passes through a concave lens?

When parallel rays of light pass through a concave lens, they diverge or spread apart. This lens is known for causing light to diverge.

4. What is the focal point, and how does it differ for concave and convex lenses?

The focal point is the point where parallel rays of light either converge or appear to diverge from after passing through the lens. For concave lenses, the focal point is on the same side as the object, while for convex lenses, it is on the opposite side.

5. How do concave and convex lenses differ in terms of image formation?

Concave lenses form virtual, upright, and diminished images for real objects placed beyond the focal point. Convex lenses form real, inverted, and magnified images for objects beyond their focal point.

Difference Between Capacitor And Inductor

Class 12

Posted by : konetwon / On : September 14, 2023

Difference Between Capacitor And Inductor: In the realm of electronics and electrical engineering, two fundamental passive components stand out: capacitors and inductors. These components play pivotal roles in shaping electrical circuits, but they do so in distinct ways.

Understanding the differences between capacitors and inductors is essential for designing and analyzing electronic circuits. In this article, we will explore the key disparities between these two components, their properties, and their applications.

Difference Between Capacitor And Inductor

Capacitors:

1. What Is a Capacitor?

A capacitor is an electronic component that stores electrical energy in the form of an electric field. It consists of two conductive plates separated by an insulating material, known as a dielectric. When a voltage is applied across the plates, electric charge accumulates on them, creating an electric field between the plates. This stored energy can be quickly released when needed.

2. Key Properties of Capacitors:

Capacity (Capacitance): Capacitance is the measure of a capacitor’s ability to store electrical charge. It is measured in farads (F).
Voltage Rating: Capacitors have a maximum voltage they can withstand before breaking down.
Charge and Discharge: Capacitors can store and release energy quickly, making them suitable for applications such as smoothing power supplies and timing circuits.
Frequency Dependency: Capacitors’ impedance (resistance to the flow of alternating current) decreases as the frequency of the signal increases.

3. Applications of Capacitors:

Filtering: Capacitors are used to filter out noise and stabilize voltage levels in power supplies.
Timing: They play a crucial role in timing circuits, oscillators, and signal delay applications.
Energy Storage: Capacitors store energy in devices like camera flashes and defibrillators.

Inductors:

1. What Is an Inductor?

An inductor is a passive electrical component that stores energy in the form of a magnetic field when current flows through it. It typically consists of a coiled wire or a wound conductor. The magnetic field generated by the current induces a voltage, opposing the change in current flow. This property is described by Faraday’s law of electromagnetic induction.

2. Key Properties of Inductors:

Inductance: Inductance is the measure of an inductor’s ability to store energy in a magnetic field. It is measured in henrys (H).
Resistance (DC): Inductors resist changes in current flow and act as a short circuit for direct current (DC).
Reactance (AC): Inductors have reactance, which is an opposition to alternating current (AC) flow, and it increases with frequency.
Energy Storage: Inductors store energy in magnetic fields and can release it gradually.

3. Applications of Inductors:

Filtering: Inductors are used in power supplies and filters to reduce high-frequency noise.
Signal Processing: They can be found in analog circuits, such as amplifiers and analog filters.
Energy Storage: Inductors are used in transformers for voltage conversion and inductors store energy in some power supplies.

Key Differences:

Storage Mechanism: Capacitors store energy in an electric field, whereas inductors store energy in a magnetic field.
Impedance: Capacitors have decreasing impedance with increasing frequency (opposite to inductors).
Reactance: Capacitors block DC and allow AC, while inductors block AC and allow DC.
Applications: Capacitors are commonly used for energy storage, filtering, and timing. Inductors are often used in signal processing, energy storage, and voltage conversion.
Physical Structure: Capacitors have two conductive plates separated by a dielectric. Inductors consist of wound coils of wire.

In summary, capacitors and inductors are crucial components in electronics, each with distinct properties and applications. Understanding their differences is essential for designing and troubleshooting electronic circuits effectively. Whether you’re building a power supply, designing an amplifier, or working on a complex electronic system, capacitors and inductors will play vital roles in shaping your circuits.

Read More

Frequently Asked Questions (FAQs) Difference Between Capacitor And Inductor

1. What is the fundamental difference between capacitors and inductors?

The fundamental difference lies in how they store energy. Capacitors store energy in an electric field, while inductors store energy in a magnetic field.

2. How is capacitance different from inductance?

Capacitance (measured in farads) is a measure of a capacitor’s ability to store electrical charge, while inductance (measured in henrys) is a measure of an inductor’s ability to store energy in a magnetic field.

3. Do capacitors and inductors behave differently with direct current (DC) and alternating current (AC)?

Yes, capacitors and inductors have opposite behaviors with DC and AC. Capacitors block DC and allow AC to pass, while inductors block AC and allow DC to pass.

4. How do capacitors and inductors affect the frequency of an AC signal?

Capacitors exhibit a decrease in impedance (resistance to the flow of AC) as the frequency increases, whereas inductors experience an increase in impedance with rising frequency.

5. What are some common applications of capacitors?

Capacitors find application in energy storage, noise filtering within power supplies, and precision timing circuits. They are also commonly incorporated into devices such as camera flashes.

Van De Graaff Generator 12th physics

Class 12

Posted by : konetwon / On : September 14, 2023

Van De Graaff Generator: The Van de Graaff generator is a remarkable device in the realm of physics and electrical engineering. It stands as a testament to the ingenuity of scientists and inventors who have pushed the boundaries of our understanding of electricity and electrostatics. Named after its creator, Robert J. Van de Graaff, this machine is a captivating instrument that generates high voltages and produces awe-inspiring electrical sparks.

Van De Graaff Generator 12th physics

1. Introduction

1.1 What is a Van de Graaff Generator?

The Van de Graaff generator is a captivating electrostatic contraption engineered for the production of high voltages. It is purpose-built to illustrate and explore the intricacies of electrostatic principles and frequently serves as an educational instrument in physics laboratories. Bearing the name of its creator, Robert J. Van de Graaff, this apparatus possesses the remarkable capability to generate mesmerizing electrical sparks and stands as a vital instrument across an array of disciplines, encompassing nuclear physics and particle acceleration.

1.2 How Does It Work?

The operation of a Van de Graaff generator is based on the principles of static electricity. It involves the continuous transfer of electric charge, typically through a moving belt, to a large hollow metal sphere. This process accumulates electric potential on the sphere, creating a high voltage difference between the sphere and the ground. As a result, the generator can produce impressive electrical sparks.

2. History of the Van de Graaff Generator

2.1 The Inventor – Robert J. Van de Graaff

The Van de Graaff gen was invented by Robert J. Van de Graaff in the early 1930s. Van de Graaff, an American physicist, developed this device while conducting research on nuclear physics at Princeton University. His goal was to create a machine capable of producing high-energy particles for use in nuclear experiments.

2.2 Development and Advancements

Over the years, Van de Graaff generators have seen significant improvements and adaptations. These generators have been upsized to accommodate various applications, such as particle accelerators and X-ray production machinery. In contemporary times, advanced iterations of the generator find extensive use in research institutions, universities, and laboratories around the globe.

3. The Components of a Van de Graaff Generator

3.1 The Generator’s Structure

A typical Van de Graaff gen consists of a metal column that supports various components. At the top of the column, there is a large metal sphere, which is one of the key elements of the generator.

3.2 The Motor

The motor, usually located at the base, drives the generator. It powers the continuous movement of the belt, which plays a critical role in charge accumulation.

3.3 The Belt

The belt in a Van de Graaff generator is typically made of an insulating material, such as rubber or a synthetic polymer. It is looped around two pulleys – one at the base and one at the top – and continuously moves in a single direction.

3.4 The Comb

Near the bottom of the generator, a small comb with sharp points is positioned close to the belt. This comb collects charges from the belt as it moves.

3.5 The Terminal

The terminal, connected to the large sphere at the top, is where the accumulated charge is stored. It can be used to discharge the high voltage or to produce electrical sparks.

4. Operation of a Van de Graaff Generator

4.1 Charging Process

The operation of a Van de Graaff gen begins with the motor turning the lower pulley, which drives the insulating belt. As the belt moves upwards, it passes near the comb at the bottom. The comb’s sharp points ionize the air and transfer electrons onto the moving belt, making it negatively charged.

4.2 The Role of Insulation

The insulating belt prevents charge from escaping and allows it to accumulate on the large sphere. Since like charges repel each other, the accumulated negative charge on the sphere repels electrons from the ground (or any nearby object), creating a strong electric field.

4.3 Maximum Voltage and Spark Length

The maximum voltage a Van de Graaff generator can produce depends on various factors, including the size of the sphere, the speed of the belt, and the surrounding conditions. When the electric field strength becomes high enough, it can ionize the surrounding air, allowing sparks to jump from the sphere to nearby objects, creating a visually stunning display of electrical discharge.

5. Applications of Van de Graaff Generators

5.1 Particle Acceleration

Van de Graaff generators find application in particle accelerators where they create and propel charged particles like protons and electrons to high energy levels. These accelerated particles play pivotal roles in nuclear physics experiments and medical applications, including cancer therapy.

5.2 Nuclear Physics Research

In the field of nuclear physics, Van de Graaff generators assume a crucial role in the examination of atomic nuclei and their characteristics. They are instrumental in producing particle beams that are directed towards target nuclei, allowing scientists to observe the resulting reactions and gather valuable data.

5.3 X-ray Production

Certain Van de Graaff generators are customized for X-ray production, a technology with diverse applications encompassing medical imaging and non-destructive testing in various industrial sectors.

5.4 Educational Demonstrations

Van de Graaff generators are frequently employed in educational environments to instruct students about the principles of electrostatics and the characteristics of electric charges. They provide captivating demonstrations of electrical phenomena.

6. Challenges and Limitations

6.1 Maintenance

Maintaining a Van de Graaff generator can be challenging, as the insulating belt may degrade over time, and mechanical components require periodic upkeep.

6.2 Safety Concerns

Due to the high voltages they can produce, Van de Graaff generators pose safety risks. Operators must take precautions to avoid electric shocks and sparks. Additionally, the discharge of high voltages can damage electronic equipment.

Conclusion

The Van de Graaff gen, an invention born out of curiosity and scientific exploration, has found its place in various fields, from fundamental physics research to medical applications. With its ability to generate high voltages and produce striking electrical displays, it continues to captivate and educate students and researchers alike. While it comes with maintenance challenges and safety considerations, its contributions to the advancement of science and technology are undeniable. Robert J. Van de Graaff’s creation continues to be a source of inspiration and innovation in the world of physics and beyond.

Read More

Frequently Asked Questions (FAQs) Van De Graaff Generator

1. What is a Van de Graaff Generator?

A Van de Graaff generator is an electrostatic apparatus created with the purpose of generating high voltages. Its functioning is based on the principles of static electricity, and it is commonly employed for educational and experimental purposes in physics laboratories.

2. Who Invented the Van de Graaff Generator?

The Van de Graaff generator was the brainchild of American physicist Robert J. Van de Graaff, conceived in the early 1930s during his research pursuits in the realm of nuclear physics at Princeton University.

3. How Does a Van de Graaff Generator Work?

A Van de Graaff gen operates by continuously transferring electric charge, usually through a moving insulating belt, to a large hollow metal sphere. This accumulation of electric potential on the sphere results in a high voltage difference between the sphere and the ground, which can produce electrical sparks.

4. What Are the Key Components of a Van de Graaff Generator?

The main components of a Van de Graaff gen include the metal sphere, the motor, the insulating belt, the comb, and the terminal. The motor drives the belt, which transfers charge to the sphere. The comb helps collect charges, and the terminal stores the accumulated charge.

5. What Are Some Applications of Van de Graaff Generators?

Van de Graaff generators find applications across a wide spectrum of fields, including particle acceleration, nuclear physics research, X-ray production, and educational demonstrations. Their unique capability to generate high-energy particles contributes to experiments and medical treatments.

Difference Between Electric Field And Magnetic Field

Class 10

Posted by : konetwon / On : September 14, 2023

Difference Between Electric Field And Magnetic Field: In the realm of electromagnetism, two fundamental concepts are at play: the electric field and the magnetic field.

These fields are essential for understanding the behavior of charged particles and the interaction between electricity and magnetism. In this article, we’ll explore the key differences between electric fields and magnetic fields and how they shape the world of physics and technology.

Difference Between Electric Field And Magnetic Field

1. Introduction

1.1 What is an Electric Field?

An electric field (E) is a spatial area surrounding an electrically charged object in which another charged object encounters a force. Electric fields arise from electric charges, whether they are positively or negatively charged, and they apply a force to other charged particles contingent upon their charge and the field’s intensity.

1.2 What is a Magnetic Field?

A magnetic field (B) is a defined region in space surrounding either a magnetic object or a moving charged particle where magnetic forces come into play. These magnetic fields originate from diverse sources, encompassing the presence of magnets, the motion of electrically charged particles, and the circulation of electric currents.

2. Nature of Fields

2.1 Electric Field:

Electric fields are produced by electric charges.
They are always present, whether or not there are other charges nearby.
Electric field lines start from positive charges and terminate at negative charges.
Electric field lines are radially outward from positive charges and radially inward toward negative charges.
Electric fields can do work on charged particles, causing them to move along the field lines.

2.2 Magnetic Field:

Magnetic fields are created through the action of magnets, the motion of electrically charged particles, and the presence of electric currents.
Magnetic fields form closed loops, with no magnetic monopoles (separate north or south poles).
They only exist in the presence of moving charges or currents.
Magnetic field lines are continuous loops, never starting or ending.
Magnetic fields can exert forces on moving charged particles but cannot do work on stationary charged particles.

3. Sources of Fields

3.1 Electric Field Sources:

Electric fields are produced by stationary electric charges, both positive and negative.
Objects that have an excess or deficit of electrons create electric fields.

3.2 Magnetic Field Sources:

Magnetic fields are generated by magnets, such as permanent magnets and electromagnets.
Moving electric charges, such as current-carrying wires, also produce magnetic fields.

4. Interaction with Charged Particles

4.1 Electric Field Interaction:

Electric fields exert forces on charged particles, causing them to accelerate or move in the direction of the field lines.
Charged particles can gain or lose electrical potential energy when moving in an electric field.

4.2 Magnetic Field Interaction:

Magnetic fields exert forces only on moving charged particles (currents).
The force experienced by a moving charged particle is perpendicular to both its velocity and the magnetic field direction.
Magnetic fields do not perform work on stationary charged particles.

5. Mathematical Representation

5.1 Electric Field Representation:

Electric fields are typically denoted and represented using vectors labeled as (E).
The electric field at a specific point is mathematically expressed as E = F/q, where F represents the force encountered by a test charge (q) positioned within that field.

5.2 Magnetic Field Representation:

Magnetic fields are represented by vectors (B).
The magnetic field at a point is given by B = (μ₀/4π) * (I x r^2), where μ₀ is the permeability of free space, I is the current, and r is the distance from the current-carrying wire.

6. Applications

6.1 Electric Field Applications:

Electric fields are essential in electronics, from powering household appliances to charging mobile devices.
Capacitors store electric energy using electric fields.
Electric fields are crucial in electrostatic applications, such as inkjet printers and photocopiers.

6.2 Magnetic Field Applications:

Magnetic fields are fundamental to the operation of electric motors and generators.
Magnetic fields are instrumental in the field of medical diagnostics, notably in magnetic resonance imaging (MRI).
Magnetic fields play a pivotal role in transportation systems such as particle accelerators and maglev trains.

Conclusion

In summary, electric fields and magnetic fields are distinct but interrelated aspects of electromagnetism. Electric fields arise from stationary electric charges and impose forces on charged particles, whereas magnetic fields originate from magnets and the movement of charges, influencing moving charged particles. Understanding these fields is pivotal in various scientific and technological domains, from electronics to medical imaging and transportation.

Read More

Frequently Asked Questions (FAQs) Difference Between Electric Field And Magnetic Field

1. What is the fundamental difference between electric fields and magnetic fields?

The primary difference is in their sources and interactions. Electric fields originate from stationary electric charges and exert forces on charged particles, while magnetic fields result from magnets, moving electric charges, or currents and affect moving charged particles.

2. Are electric fields and magnetic fields always present together?

Not necessarily. Electric fields can exist independently of magnetic fields and vice versa. They are distinct phenomena, although they often interact in certain situations, such as in electromagnetic waves.

3. How do electric and magnetic fields interact in electromagnetic waves?

In electromagnetic waves, changing electric fields create changing magnetic fields, and vice versa. This interplay allows for the propagation of electromagnetic radiation, such as light and radio waves.

4. Do electric fields and magnetic fields have the same units of measurement?

Certainly, electric fields and magnetic fields utilize separate units of measurement. Electric fields are denoted in volts per meter (V/m), while magnetic fields are conventionally measured in units of teslas (T) or gauss (G).

5. Can magnetic fields exert forces on stationary charged particles?

No, magnetic fields do not exert forces on stationary charged particles. They only affect moving charged particles due to their motion.

Dielectric Polarization In Polar And Nonpolar Material And Dielectric Constant

Class 12

Posted by : konetwon / On : September 14, 2023

Dielectric Polarization In Polar And Nonpolar Material And Dielectric Constant: In the world of electromagnetism and materials science, the concept of dielectric polarization plays a central role.

It is a phenomenon that occurs when electric charges within a material shift and create an electric dipole moment, resulting in various intriguing effects. In this article, we will delve into dielectric polarization, exploring its occurrence in both polar and nonpolar materials, and discuss the important parameter known as the dielectric constant.

Dielectric Polarization In Polar And Nonpolar Material And Dielectric Constant

1. Understanding Dielectric Polarization

1.1 What is Dielectric Polarization?

Dielectric polarization is the result of applying an electric field to a material, prompting the movement of its electric charges, which typically involve electrons and nuclei, from their original equilibrium positions. This movement leads to the formation of electric dipoles within the material, wherein positive and negative charges become separated, while the material itself maintains an overall electrical neutrality. The induced dipoles align with the electric field, and this alignment contributes to the material’s response to the applied field.

1.2 Electric Dipoles

An electric dipole consists of two equal and opposite electric charges of magnitude, +q and -q, separated by a distance, denoted as “d.” The electric dipole moment, represented by the vector “p,” is defined as the product of the charge and the distance between them: p = qd. The direction of the dipole moment points from the negative charge to the positive charge.

1.3 Dielectric Polarization Mechanisms

Dielectric polarization can occur through several mechanisms, including:

Electronic Polarization: In polar materials, such as water, the electron cloud around atoms or molecules shifts under the influence of an external electric field.
Ionic Polarization: In ionic solids, like table salt (sodium chloride), positive and negative ions are displaced within the crystal lattice.
Orientational Polarization: In some materials, like ferroelectric crystals, the entire molecules or ions can rotate in response to an electric field.

2. Dielectric Polarization in Polar Materials

2.1 Polar and Nonpolar Materials

Materials can be broadly categorized as polar or nonpolar based on their molecular structure and symmetry.

Polar Materials: These materials have an uneven distribution of charge due to an asymmetrical arrangement of atoms or molecules. Examples include water (H2O) and hydrogen fluoride (HF).
Nonpolar Materials: These materials have a symmetrical charge distribution, resulting in no net dipole moment. Examples include oxygen (O2) and nitrogen (N2).

2.2 Dielectric Polarization in Polar Materials

Polar materials exhibit prominent dielectric polarization because of their inherent dipole moments. When an external electric field is imposed, these dipoles align with the field’s direction, amplifying the material’s dielectric polarization. Consequently, polar materials typically possess a higher dielectric constant (ε) in comparison to nonpolar materials.

3. Dielectric Polarization in Nonpolar Materials

3.1 Dielectric Polarization in Nonpolar Materials

In nonpolar materials, dielectric polarization still occurs, but it is typically weaker compared to polar materials. In these materials, electrons within atoms and molecules are displaced, albeit to a smaller extent. While the induced dipoles are less pronounced, they still contribute to the overall dielectric polarization.

3.2 Effect of Temperature

The degree of dielectric polarization in nonpolar materials can be influenced by temperature. As temperature increases, thermal motion disrupts the alignment of induced dipoles, leading to a decrease in dielectric constant.

4. Dielectric Constant (Relative Permittivity)

4.1 Definition and Significance

The dielectric constant, often denoted as ε or εr (relative permittivity), is a dimensionless quantity that represents how effectively a material can store electrical energy in electric field. It is a crucial parameter in understanding the behavior of dielectric materials.

The dielectric constant is defined as the ratio of the electric field (E) in a vacuum (or air) to the electric field (E) within the dielectric material when the same voltage is applied. Mathematically, it is expressed as:

$ε = E ^{vacuum}$

The dielectric constant provides insight into the material’s ability to store electrical energy by forming induced dipoles. Higher dielectric constants indicate better dielectric properties, as they signify a more effective response to an applied electric field.

4.2 How Dielectric Constant is Measured

Dielectric constant measurements involve placing a dielectric material between two conductive plates and applying a known voltage across them. The resulting electric field within the material is compared to the field in a vacuum or air. This comparison allows for the calculation of the dielectric constant using the formula mentioned earlier.

Dielectric constant values vary widely among different materials. For example, the dielectric constant of a vacuum is defined as exactly 1, while common dielectric materials like water have higher values (around 78.5 at room temperature).

4.3 Influence on Capacitance

The dielectric constant plays a pivotal role in the design and operation of capacitors. A capacitor consists of two conductive plates separated by a dielectric material. The capacitance (C) of the capacitor is directly proportional to the dielectric constant (ε) and the surface area of the plates (A) and inversely proportional to the distance between the plates (d):

$C = ε A/d$

By using dielectric materials with higher dielectric constants, the capacitance of a capacitor can be significantly increased, allowing it to store more electrical charge for a given voltage.

5. Applications of Dielectric Polarization

5.1 Capacitors

One of the most common applications of dielectric polarization is in capacitors. Capacitors are essential electronic components used in a wide range of devices, including radios, computers, and power supplies. Dielectric materials are placed between the capacitor plates to enhance capacitance, which, in turn, affects the device’s energy storage and discharge characteristics.

5.2 Dielectric Resonators

Dielectric resonators are utilized in microwave and radio frequency (RF) applications as components that selectively filter specific frequencies. The dielectric properties of these resonators, including their dielectric constant and loss tangent, determine their performance in various communication and radar systems.

5.3 Insulators and Electrical Conductors

Dielectric materials, both polar and nonpolar, serve as insulators in electrical and electronic systems. They prevent the flow of electric current and are essential for electrical safety. In contrast, conductive materials, renowned for their low dielectric constants, facilitate efficient electrical conduction and are extensively applied in electrical wiring and circuits.

6. Future Developments in Dielectric Materials

In recent years, researchers have been actively exploring new dielectric materials and pushing the boundaries of dielectric performance. Some promising areas of development include:

6.1 High-K Dielectrics:

Materials with high dielectric constants (K values) are of interest because they can lead to smaller and more energy-efficient electronic devices. Innovations in high-K dielectrics have the potential to revolutionize the semiconductor industry.

6.2 Organic Dielectrics:

Researchers are exploring organic materials as dielectric substances due to their affordability, flexibility, and promising suitability for applications in the realm of organic electronics. Organic dielectrics are important in the development of flexible displays, organic solar cells, and thin-film transistors.

6.3 Ferroelectric and Multiferroic Materials:

Ferroelectric materials, exemplified by substances like lead zirconate titanate (PZT), display inherent spontaneous polarization and find utility in diverse applications, including non-volatile memory devices. Multiferroic materials, which possess both ferroelectric and ferromagnetic properties, are also under exploration for advanced technologies.

6.4 Dielectric Metamaterials:

Researchers are currently investigating metamaterials designed to possess distinctive dielectric characteristics for potential applications in optics, electromagnetic cloaking, and superlensing.

6.5 Energy Storage:

Dielectric materials are being studied for their potential use in energy storage applications, including dielectric capacitors for high-energy density storage and energy harvesting devices.

Conclusion

Dielectric polarization is a fundamental phenomenon that shapes the behavior of materials in response to electric fields. Whether in polar or nonpolar materials, dielectric polarization influences the properties of materials and finds applications in various fields, from electronics to energy storage.

Read More

Frequently Asked Questions (FAQs) Dielectric Polarization In Polar And Nonpolar Material And Dielectric Constant

1. What is dielectric polarization?

Dielectric polarization is the phenomenon where electric charges within a material shift and create electric dipoles in response to an applied electric field. It results in the alignment of electric dipoles within the material.

2. What are electric dipoles?

Electric dipoles consist of two equal and opposite electric charges separated by a distance. They create a net dipole moment, represented by a vector pointing from the negative charge to the positive charge.

3. How does dielectric polarization occur in materials?

Dielectric polarization can occur through various mechanisms, including electronic polarization (electron cloud shift), ionic polarization (displacement of ions), and orientational polarization (rotation of molecules or ions).

4. What are polar and nonpolar materials?

Polar materials have an uneven distribution of charge due to an asymmetrical arrangement of atoms or molecules. Nonpolar materials have a symmetrical charge distribution, resulting in no net dipole moment.

5. Does dielectric polarization exhibit greater strength in polar materials when compared to nonpolar materials?

Yes, dielectric polarization is typically stronger in polar materials because they already possess inherent dipole moments due to their asymmetrical structure.

Periodic Table Class 11

Class 11

Posted by : konetwon / On : September 14, 2023

Periodic Table Class 11: The periodic table is one of the foundational pillars of chemistry, and for Class 11 students embarking on their chemistry journey, it is a crucial topic to understand.

This comprehensive guide aims to provide Class 11 students with an in-depth exploration of the periodic table, covering its history, organization, periodic trends, and the significance of this iconic arrangement of elements.

Periodic Table Class 11

1. The Historical Journey of the Periodic Table

1.1 The Early Days of Chemistry:

The study of elements and their properties dates back to ancient times, with philosophers like Aristotle contemplating the nature of substances.
Alchemists in the Middle Ages contributed to the understanding of materials, although their methods were often mystical.

1.2 Mendeleev’s Periodic Table:

Dmitri Mendeleev, a Russian chemist, is credited with creating the first periodic table in 1869.
Mendeleev organized elements based on their atomic mass, noticing that elements with similar properties occurred at regular intervals.
He left gaps for undiscovered elements and accurately predicted their properties.

1.3 The Modern Periodic Table:

The modern periodic table is organized by increasing atomic number, which is the number of protons in an atom’s nucleus.
Elements are arranged into periods (horizontal rows) and groups (vertical columns).
The periodic table accommodates all known elements, including synthetic ones.

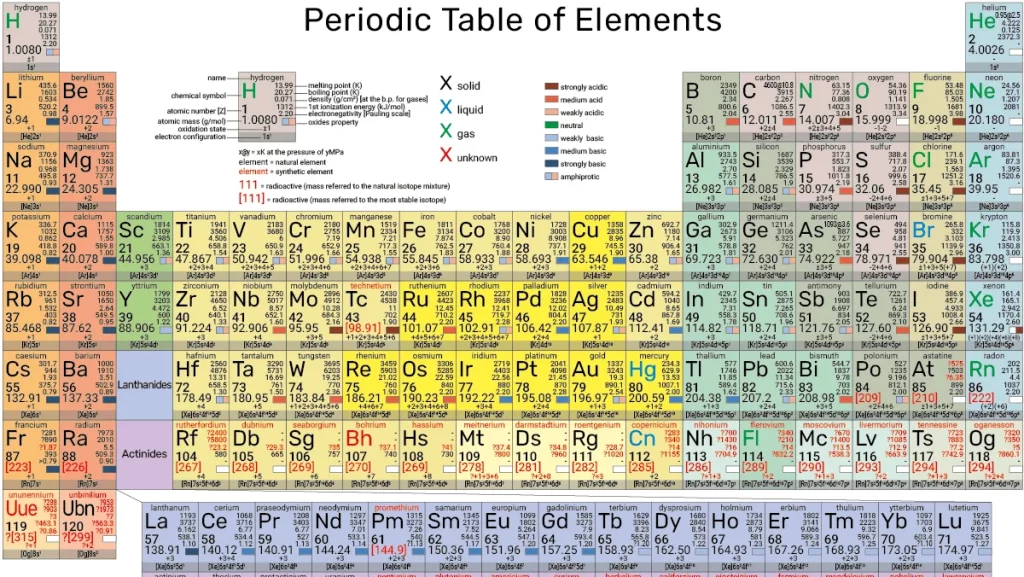Periodic Table

2.1 Periods and Groups:

A period is a horizontal row on the periodic table, and there are seven periods in total.
A group (or family) is a vertical column, and there are 18 groups.
Elements in the same group share similar chemical properties due to their similar electron configurations.

2.2 Atomic Number and Atomic Mass:

The atomic number of an element is its unique identifier, representing the number of protons in its nucleus.
The atomic mass (or atomic weight) is the average mass of an element’s isotopes.

2.3 Electronic Configuration:

The arrangement of electrons in an atom’s energy levels determines its chemical behavior.
Understanding electronic configurations helps predict how elements will bond and react.

3. Elements and Their Properties

3.1 Metals, Non-Metals, and Metalloids:

The periodic table can be divided into three main categories: metals, non-metals, and metalloids.
Metals are typically shiny, conductive, and have high melting and boiling points.
Non-metals are often dull, poor conductors, and have lower melting and boiling points.
Metalloids exhibit properties of both metals and non-metals.

3.2 Representative and Transition Elements:

Representative elements are found in the “s” and “p” blocks of the periodic table.
Transition elements are located in the “d” and “f” blocks and are known for their variable oxidation states and colorful compounds.

4. Periodic Trends

4.1 Atomic Radius:

Atomic radius is the size of an atom and decreases across a period and increases down a group.
This trend is due to the effective nuclear charge and the number of electron energy levels.

4.2 Ionization Energy:

Ionization energy is the energy required to remove an electron from an atom.
It increases across a period and decreases down a group due to electron shielding and effective nuclear charge.

4.3 Electronegativity:

Electronegativity is an element’s ability to attract electrons in a chemical bond.
It increases across a period and decreases down a group.

4.4 Electron Affinity:

Electron affinity is the energy change when an atom gains an electron.
It generally increases across a period and decreases down a group.

5. The Significance of the Periodic Table

5.1 Predicting Element Properties:

The periodic table allows scientists to predict the properties of elements, even those not yet discovered.
It provides insights into an element’s reactivity, bonding behavior, and physical characteristics.

5.2 Understanding Chemical Bonding:

The arrangement of elements in the periodic table explains how chemical bonds form.
Elements in the same group often share similar bonding tendencies.

5.3 Analyzing Chemical Reactions:

The periodic table helps chemists understand and predict the outcomes of chemical reactions.
It guides reaction stoichiometry and the formation of products.

5.4 Applications in Real Life:

The periodic table has practical applications in various fields, from materials science and medicine to environmental science and energy production.
It is essential for designing new materials and understanding their properties.

6. Key Concepts and Practical Tips for Class 11 Students

As you study the periodic table in your Class 11 chemistry curriculum, here are some key concepts and practical tips to help you grasp this fundamental topic effectively:

6.1 Understand Periods and Groups:

Pay close attention to the structure of the periodic table, with its rows (periods) and columns (groups).
Recognize that elements in the same group have similar properties due to their electron configurations.

6.2 Atomic Number vs. Atomic Mass:

Differentiate between the atomic number (Z) and atomic mass (A) of elements.
The atomic number defines the element, while the atomic mass accounts for isotopes.

6.3 Electronic Configurations:

Learn how to write electronic configurations for elements, as they play a crucial role in understanding reactivity and bonding.

6.4 Periodic Trends:

Master the periodic trends of atomic radius, ionization energy, electronegativity, and electron affinity.
Recognize how these trends change across periods and down groups.

6.5 Chemical Bonding:

Understand how elements’ positions in the periodic table influence the type of chemical bonds they form.
This knowledge is vital for predicting bond types and reactions.

6.6 Practice Problem-Solving:

Solve a variety of problems related to the periodic table to reinforce your understanding.
Work on exercises that involve predicting element behavior and calculating trends.

6.7 Real-World Applications:

Explore the practical applications of the periodic table in various fields.
Consider how elements are used in everyday life, technology, and industry.

7. Resources for Further Learning

To deepen your understanding of the periodic table, consider using additional resources:

Textbooks: Consult your Class 11 chemistry textbook for in-depth explanations and practice problems related to the periodic table.
Online Tutorials: Explore online chemistry tutorials and video lessons that provide visual explanations and demonstrations.
Interactive Periodic Tables: Use interactive periodic tables available online or through chemistry apps. These tools allow you to explore element properties and trends interactively.
Periodic Table Games: Engage in educational games and quizzes that make learning about the periodic table fun and interactive.
Chemistry Forums: Join online chemistry forums or communities where you can ask questions, share knowledge, and learn from fellow students and experts.
Laboratory Experiments: If possible, participate in chemistry laboratory experiments that involve elements from the periodic table. Hands-on experience can enhance your understanding.

8. Study Strategies for Success

To excel in your Class 11 chemistry studies, particularly when delving into the periodic table, consider the following study strategies:

8.1 Consistent Practice:

Regularly review and practice the concepts related to the periodic table. Consistency is key to mastering this fundamental topic.

8.2 Flashcards:

Create flashcards with element names, symbols, atomic numbers, and key properties. Use these flashcards for quick and effective revision.

8.3 Group Study:

Collaborate with classmates for group study sessions. Discussing and teaching each other can reinforce your understanding.

8.4 Mnemonics:

Use mnemonics or memory aids to remember the order of elements in a group or other important periodic table information.

8.5 Visual Aids:

Utilize visual aids, such as color-coded periodic tables and diagrams, to help you grasp the organization and trends effectively.

8.6 Online Resources:

Explore online resources, including educational websites, interactive periodic tables, and YouTube tutorials that offer alternative explanations and examples.

8.7 Regular Review:

Periodically revisit previously learned concepts to ensure retention. This can be particularly useful for mastering periodic trends.

9. Realizing the Beauty of Chemistry

While the periodic table may seem like a static chart of elements, it holds the key to understanding the dynamic world of chemistry. As you progress through your Class 11 chemistry course, you’ll come to appreciate how this elegant arrangement of elements simplifies complex chemical phenomena.

Moreover, the periodic table transcends classroom boundaries; it’s a universal language understood by scientists worldwide. It’s a testament to human ingenuity and the power of collaboration among scientists over centuries.

Remember, the periodic table is not merely a chart—it’s a gateway to exploring the mysteries of matter, the wonders of chemical reactions, and the boundless possibilities of science. Embrace your journey into the world of chemistry, and let the periodic table be your guide.

Your curiosity and dedication will unlock a realm of knowledge that will not only enrich your academic pursuits but also deepen your appreciation for the beauty of the natural world. Happy studying!

10. Classroom and Beyond

As you progress through your Class 11 chemistry curriculum and explore the periodic table, keep in mind that chemistry extends far beyond the classroom. Here are some ways to engage with chemistry in your everyday life:

10.1 Observing the Elements:

Look around you and identify elements in your environment. Consider the metals used in your electronics, the gases in the air you breathe, and the compounds in household items.

10.2 Chemistry in Nature:

Explore the chemistry of the natural world. Learn about the chemical processes that occur in plants, animals, and geological formations.

10.3 Chemistry in Technology:

Investigate how chemistry drives technological advancements. Discover how elements and compounds are used in cutting-edge innovations.

10.4 Chemistry in Medicine:

Delve into the role of chemistry in healthcare. Learn about pharmaceuticals, medical diagnostics, and the chemistry behind disease prevention and treatment.

10.5 Environmental Chemistry:

Explore the impact of human activities on the environment and the role of chemistry in addressing environmental challenges.

10.6 Chemistry in Art and Culture:

Appreciate the connection between chemistry and art, from pigments used in paintings to the chemistry of cuisine.

12. Seek Guidance and Stay Curious

Remember that chemistry, including the pd table, can be a challenging subject at times, but it’s also incredibly rewarding. Don’t hesitate to seek guidance from your teachers, classmates, or online resources when you encounter difficulties. Chemistry is a subject that rewards curiosity and perseverance.

Stay curious and inquisitive. Ask questions, conduct experiments if possible, and explore chemistry-related topics that pique your interest. The pd table is just the beginning of a fascinating journey into the world of chemistry, where countless discoveries await.

As you continue your studies in Class 11 and beyond, keep in mind that chemistry is not confined to textbooks and classrooms. It’s a vibrant field that impacts every aspect of our lives. Whether you aspire to be a scientist, engineer, healthcare professional, or simply a curious learner, chemistry will provide you with valuable insights and a deeper understanding of the world around you.

Embrace the pd table as your roadmap, and let your curiosity drive your exploration of the captivating realm of chemistry. With dedication and a thirst for knowledge, you’ll find that chemistry opens doors to endless possibilities and a lifelong appreciation for the wonders of the natural world. Enjoy your journey into the world of chemistry!

The pd table is a foundational concept in chemistry that serves as a roadmap to the world of elements and their properties. As a Class 11 student, your journey through chemistry will be greatly enriched by a solid grasp of the pd table and its associated principles. Embrace the pd table as a tool that will empower you to unravel the mysteries of matter, and enjoy the adventure of discovery as you explore the fascinating world of chemistry.

Conclusion

Read More

Frequently Asked Questions (FAQs) Periodic Table

1. What is the periodic table?

The pd table is a tabular arrangement of chemical elements, organized by their atomic number, which represents the number of protons in an atom’s nucleus. It groups elements with similar properties in columns called groups or families.

2. Who developed the first periodic table, and when was it created?

The first pd table was developed by Dmitri Mendeleev, a Russian chemist, in 1869. He organized elements based on their atomic masses and predicted the properties of undiscovered elements.

3. How are elements arranged in the periodic table?

Elements are arranged by increasing atomic number from left to right and top to bottom. They are grouped into periods (rows) and groups (columns) based on similar electronic configurations and properties.

4. What are periods and groups in the periodic table?

Periods are the horizontal rows in the pd table, numbered from 1 to 7. Groups, also known as families, are the vertical columns, numbered from 1 to 18.

5. What are representative elements and transition elements?

Representative elements, also called main group elements, are found in the “s” and “p” blocks of the pd table. Transition elements are located in the “d” and “f” blocks.

Molecular Mass Of Acetic Acid

Class 9

Posted by : konetwon / On : September 14, 2023

Molecular Mass Of Acetic Acid: In the realm of organic chemistry, understanding the molecular mass of compounds is a fundamental concept.

Molecular mass, also known as molecular weight or molar mass, plays a pivotal role in various chemical calculations and analyses. In this article, we will delve into the molecular mass of acetic acid (CH3COOH), a compound that is not only vital in the laboratory but also widely recognized in everyday life.

Molecular Mass Of Acetic Acid

Acetic Acid (CH3COOH): The Basics

Before we dive into the molecular mass of acetic acid, let’s get to know the compound itself. Acetic acid, chemically represented as CH3COOH, is a simple organic acid with a distinctive pungent odor and sour taste. It is the primary component responsible for the characteristic taste and smell of vinegar.

Acetic acid is both naturally occurring and synthetically produced. In the natural environment, acetic acid is produced via the fermentation of sugars and carbohydrates by specific bacterial strains. In controlled laboratory conditions, it can be synthetically created through diverse chemical processes.

Calculating the Molecular Mass

The molecular mass of a compound is the sum of the atomic masses of all the atoms present in a single molecule of that compound. To calculate the molecular mass of acetic acid (CH3COOH), we need to consider the atomic masses of its constituent elements: carbon (C), hydrogen (H), and oxygen (O).

Here are the approximate atomic masses of these elements:

Carbon (C): Approximately 12.01 g/mol
Hydrogen (H): Approximately 1.008 g/mol
Oxygen (O): Approximately 16.00 g/mol

Now, let’s calculate the molecular mass of acetic acid:

Mole Mass (CH3COOH) = (Number of C atoms × Atomic Weight(C)) + (Number of H atoms × Atomic Weight(H)) + (Number of O atoms × Atomic Weight(O))

In the case of acetic acid, there are 2 carbon atoms (C), 4 hydrogen atoms (H), and 2 oxygen atoms (O) in a single molecule:

Mole Mass (CH3COOH) = (2 × 12.01 g/mol) + (4 × 1.008 g/mol) + (2 × 16.00 g/mol)

Mole Mass (CH3COOH) ≈ 60.05 g/mol

Therefore, the mole mass of acetic acid (CH3COOH) is approximately 60.05 grams per mole.

Significance of Molecular Mass

The mole mass of acetic acid, like that of any compound, is significant for several reasons:

Stoichiometry: Mole mass is crucial in balancing chemical equations and determining the quantitative relationships between reactants and products in chemical reactions.
Concentration Calculations: It aids in calculating the concentration of acetic acid in solutions, a vital aspect of analytical chemistry and laboratory work.
Molar Mass: Molecular mass is used to calculate the molar mass of acetic acid, which is essential for determining the amount of acetic acid in moles.
Physical Properties: Molecular mass influences the physical properties of acetic acid, including its density, melting point, and boiling point.

Practical Applications of Acetic Acid

Acetic acid is a versatile compound with numerous practical applications:

Food Industry: It finds application as a food preservative and serves as the primary ingredient in vinegar, imparting the distinctive flavor and aroma associated with this condiment.
Chemical Industry: Acetic acid serves as a precursor in the manufacturing of numerous chemicals, among them acetic anhydride, a critical component in the synthesis of aspirin and various other pharmaceutical compounds.
Laboratory: It is employed as a reagent in chemical laboratories for various experiments and analyses.
Textile Industry: In textile manufacturing, acetic acid is used in dyeing processes.
Cleaning Products: It is found in household cleaning products like window cleaners.

In conclusion, the mole mass of acetic acid (CH3COOH) is approximately 60.05 grams per mole. This value serves as a crucial parameter in understanding the composition of acetic acid and its role in a wide range of applications, from culinary delights to industrial processes and scientific research. Acetic acid’s molecular mass is a cornerstone in the world of organic chemistry, and its versatility makes it an essential compound in various aspects of our lives.

Read More

Frequently Asked Questions (FAQs) Molecular Mass Of Acetic Acid

1. What is the molecular mass of acetic acid (CH3COOH)?

The mole mass of acetic acid is approximately 60.05 grams per mole (g/mol).

2. Why is knowing the molecular mass of acetic acid important in chemistry?

The mole mass is essential in chemistry because it helps determine the compound’s mass and composition, enabling various calculations, including stoichiometry, concentration determination, and molar mass calculations.

3. What are the constituent elements of acetic acid, and what are their atomic weights?

Acetic acid (CH3COOH) contains three elements: carbon (C), hydrogen (H), and oxygen (O). Their approximate atomic weights are:

Carbon (C): ~12.01 g/mol
Hydrogen (H): ~1.008 g/mol
Oxygen (O): ~16.00 g/mol

4. What is the method for determining the molecular mass of acetic acid?

The mole mass of acetic acid is calculated by summing the atomic masses of its constituent elements (C, H, and O) in one molecule. The calculation is as follows: Molecular Mass (CH3COOH) = (Number of C atoms × Atomic Weight(C)) + (Number of H atoms × Atomic Weight(H)) + (Number of O atoms × Atomic Weight(O))

5. What are some practical applications of acetic acid?

Acetic acid has a wide range of applications, including its use as a food preservative (vinegar), a precursor in pharmaceutical production, a reagent in chemical laboratories, a dyeing agent in the textile industry, and a component in cleaning products.

Molecular Weight Of Calcium Carbonate

Class 9

Posted by : konetwon / On : September 14, 2023

Molecular Weight Of Calcium Carbonate: In the world of chemistry, the molecular weight of a compound is a fundamental concept that underpins various aspects of chemical analysis and research.

One compound that serves as an excellent illustration of this concept is calcium carbonate (CaCO3). In this article, we will explore the molecular weight of calcium carbonate and its significance in chemistry and everyday life.

Molecular Weight Of Calcium Carbonate

Calcium Carbonate (CaCO3): The Basics

Before delving into the molecular weight of calcium carbonate, let’s acquaint ourselves with this compound. Calcium carbonate, represented chemically as CaCO3, is a common chemical compound with widespread applications in various fields.

In its native state, calcium carbonate is present in geological formations and minerals like limestone, marble, and chalk. For centuries, these naturally formed deposits have been tapped into for a wide range of purposes, spanning from construction and artistic creations to medicinal applications. Calcium carbonate is also a major component of seashells and pearls.

Understanding Molecular Weight

The molecular weight of a compound is a numerical value that reflects the mass of one mole of that compound. It can be denoted in units such as atomic mass units (amu) or grams per mole (g/mol). To calculate the molecular weight of calcium carbonate, we need to consider the atomic weights of its constituent elements: calcium (Ca), carbon (C), and oxygen (O).

Here are the atomic weights of these elements:

Calcium (Ca): Approximately 40.08 g/mol
Carbon (C): Approximately 12.01 g/mol
Oxygen (O): Approximately 16.00 g/mol

Now, we can calculate the molecular weight of calcium carbonate (CaCO3):

Molecular Weight (CaCO3) = Atomic Weight(Ca) + Atomic Weight(C) + 3 × Atomic Weight(O) Molecular Weight (CaCO3) = 40.08 g/mol + 12.01 g/mol + 3 × 16.00 g/mol Molecular Weight (CaCO3) ≈ 100.09 g/mol

Therefore, the mole weight of calcium carbonate is approximately 100.09 grams per mole.

Significance of Molecular Weight

Understanding the mole weight of a compound, such as calcium carbonate, has several practical implications:

Stoichiometry: Mole weight plays a crucial role in balancing chemical equations and determining the proportions of substances involved in chemical reactions.
Concentration Calculations: It is essential for calculating the concentration of calcium carbonate in solutions, which is significant in fields like environmental science and chemistry.
Molar Mass: Mole weight is used to calculate the molar mass of calcium carbonate, which is useful for quantifying amounts in moles.
Formulation: In various industries, including agriculture and pharmaceuticals, knowledge of molecular weight aids in formulating products and determining dosages.
Physical Properties: Molecular weight influences the physical properties of a substance, such as its density and melting and boiling points.

Practical Applications of Calcium Carbonate

Calcium carbonate has a wide range of practical applications. It is used as a dietary supplement, in the paper industry as a filler and coating pigment, in the production of cement and concrete, and as an antacid for heartburn relief. Additionally, it serves as a key component in the manufacturing of ceramics, paints, plastics, and various building materials.

In conclusion, the mole weight of calcium carbonate (CaCO3) is approximately 100.09 grams per mole. This value is not only essential in understanding the composition of calcium carbonate but also pivotal in various scientific, industrial, and everyday applications. Whether you’re studying chemical reactions, determining concentrations, or manufacturing everyday products, the concept of molecular weight remains a cornerstone in the world of chemistry and materials science.

Read More

Frequently Asked Questions (FAQs) Molecular Weight Of Calcium Carbonate

1. What is the molecular weight of calcium carbonate (CaCO3)?

The molecular weight of calcium carbonate (CaCO3) is approximately 100.09 grams per mole.

2. Why is the molecular weight of calcium carbonate important in chemistry?

The molecular weight is crucial in chemistry as it provides information about the compound’s mass and composition, which is essential for various calculations, including stoichiometry, concentration determination, and molar mass calculations.

3. What are the constituent elements of calcium carbonate, and what are their atomic weights?

Calcium carbonate consists of three elements: calcium (Ca), carbon (C), and oxygen (O). Here are their approximate atomic weights:

Calcium (Ca): ~40.08 g/mol
Carbon (C): ~12.01 g/mol
Oxygen (O): ~16.00 g/mol

4. How is the molecular weight of calcium carbonate calculated?

The mole weight of calcium carbonate is calculated by summing the atomic weights of its constituent elements: Ca, C, and three O atoms. The calculation is as follows: Molecular Weight (CaCO3) = Atomic Weight(Ca) + Atomic Weight(C) + 3 × Atomic Weight(O)

5. What are some practical applications of calcium carbonate?

Calcium carbonate finds applications across a multitude of industries, encompassing agriculture (as a soil conditioner), pharmaceuticals (as a dietary supplement), construction (in the production of cement and concrete), paper manufacturing (as a filler), and serving as an antacid for relieving heartburn.

Previous 1 2 3 4 5 6 … 18 Next