Blog
Difference Between Alternator And Generator
Difference Between Alternator And Generator: Alternators and generators are both devices that convert mechanical energy into electrical energy, but they operate on different principles and have distinct characteristics. Here’s a comparison of the key differences between alternators and generators:
Difference Between Alternator And Generator
1. Operating Principle:
- Alternator: An alternator generates electricity using the principle of electromagnetic induction. It produces alternating current (AC), where the direction of current flow continuously reverses at a specific frequency.
- Generator: A generator, often referred to as a dynamo or DC generator, produces direct current (DC) through electromagnetic induction. It typically uses a commutator to convert alternating voltage into direct current.
2. Type of Current Produced:
- Alternator: Produces alternating current (AC), which periodically changes direction. The voltage and current alternate in polarity and direction.
- Generator: Produces direct current (DC), where the voltage and current flow consistently in one direction.
3. Output Voltage:
- Alternator: Alternators usually produce higher voltages (AC) suitable for many electrical applications. Voltage regulation is typically easier in alternators.
- Generator: Generators typically produce lower voltages (DC) and require additional components like voltage regulators to achieve stable output.
4. Maintenance:
- Alternator: Alternators generally require less maintenance compared to generators. They have fewer moving parts and do not have brushes and commutators, which wear out over time.
- Generator: Generators may require more maintenance due to the brushes and commutators that need periodic replacement. This maintenance can be more demanding and costly.
5. Efficiency:
- Alternator: Alternators are generally more efficient in converting mechanical energy into electrical energy. They are commonly used in modern vehicles due to their efficiency.
- Generator: Generators may have lower efficiency due to the energy losses associated with the commutator and the conversion of AC to DC.
6. Applications:
- Alternator: Alternators are widely used in automobiles to charge the battery and power electrical systems. They are also used in power generation stations to produce AC electricity.
- Generator: Generators are often found in smaller, portable applications, such as backup power sources, construction sites, and older electrical systems that require DC power.
7. Output Frequency:
- Alternator: Alternators produce AC with a specific frequency, usually 50 or 60 Hz, depending on the application and location.
- Generator: Generators do not have a fixed frequency; the output frequency depends on the rotational speed and design of the generator.
In summary, the primary difference between alternators and generators lies in the type of current they produce (AC vs. DC) and their operating principles. Alternators generate AC and are more efficient, while generators produce DC and may require more maintenance. The choice between them depends on the specific requirements of the application.
Read More
- Physical & chemical properties of water
- Difference Between Fuse And Circuit Breaker
- Difference Between Electromagnet And Permanent Magnet
- Blind Visually Impaired Optical Low Vision Aids
- Bar Magnet As An Equivalent Solenoid
Frequently Asked Questions (FAQs) Difference Between Alternator And Generator
1. What is the fundamental difference between an alternator and a generator?
The main difference lies in the type of current they produce. An alternator generates alternating current (AC), while a generator produces direct current (DC).
2. How do alternators and generators operate differently?
Alternators work on the principle of electromagnetic induction, producing AC through changing magnetic fields. Generators also use electromagnetic induction but typically employ a commutator to convert AC to DC.
3. Can you explain the output voltage differences between alternators and generators?
Alternators generally produce higher-voltage AC, while generators typically yield lower-voltage DC. Alternators may have built-in voltage regulation, making their output more stable.
4. Which one is easier to maintain, an alternator or a generator?
Alternators are generally easier to maintain because they have fewer moving parts and do not require components like brushes and commutators, which can wear out.
5. In terms of efficiency, which is better, an alternator or a generator?
Alternators are typically more efficient in converting mechanical energy into electrical energy compared to generators, which may have energy losses due to the commutator and AC-to-DC conversion.
Physical & chemical properties of water
Physical & chemical properties of water: Water is an extraordinary substance that is essential for life as we know it. Its unique physical and chemical properties make it a universal solvent, a critical component of biological processes, and a vital resource for our planet.
In this article, we will delve into the fascinating world of water and explore its distinct physical and chemical properties.

Physical & chemical properties of water
I. Physical Properties of Water
1. State of Matter:
- Water exists in all three states of matter under normal conditions. It can be found as a solid (ice), liquid (water), or gas (water vapor) depending on temperature and pressure.
2. High Heat Capacity:
- Water has a high specific heat capacity, meaning it can absorb and store a significant amount of heat energy without a substantial temperature change. This property helps regulate Earth’s temperature and climate.
3. High Heat of Vaporization:
- Water has a high heat of vaporization, requiring a considerable amount of energy to change from a liquid to a gas. This property is why sweating cools the body.
4. Density Anomaly:
- Water’s density decreases as it freezes, causing ice to float on water. This anomaly is vital for aquatic life, as it insulates bodies of water during freezing temperatures.
5. Cohesion and Adhesion:
- Water molecules exhibit strong cohesive forces, allowing them to stick together, and adhesive forces, allowing them to adhere to other surfaces. This property is responsible for capillary action in plants and the meniscus in a glass of water.
II. Chemical Properties of Water
1. Universal Solvent:
- Water is often referred to as the “universal solvent” because of its ability to dissolve a wide range of substances, including salts, acids, bases, and polar molecules. This property is crucial for chemical reactions in living organisms and natural processes.
2. Polarity:
- Water is a polar molecule, with an uneven distribution of electron density. This results in a positive and a negative end (dipole moment), making it an excellent solvent for polar compounds.
3. Hydrogen Bonding:
- Hydrogen bonding occurs between water molecules due to the attraction between the positively charged hydrogen atoms and the negatively charged oxygen atoms. This property gives water its high boiling point, surface tension, and adhesive properties.
4. Chemical Reactivity:
- Water can act as both an acid and a base (amphoteric), participating in chemical reactions as a proton donor (acid) or acceptor (base). It plays a crucial role in various chemical reactions, such as hydrolysis and ionization.
5. Ionization:
- Water molecules can ionize into hydronium ions (H3O+) and hydroxide ions (OH-) through autoionization. This process forms the basis of the pH scale and is fundamental to the chemistry of aqueous solutions.
Conclusion
Water’s physical and chemical properties are truly remarkable and underpin its significance in both the natural world and our daily lives. Its versatility as a solvent, its ability to moderate temperature, and its essential role in biochemical processes make water a precious resource. Understanding the unique properties of water is essential for fields ranging from biology and chemistry to environmental science and engineering.
Read More
- Difference Between Fuse And Circuit Breaker
- Difference Between Electromagnet And Permanent Magnet
- Blind Visually Impaired Optical Low Vision Aids
- Bar Magnet As An Equivalent Solenoid
- Equivalent Weight of KMnO4
Frequently Asked Questions (FAQs) Physical & chemical properties of water
1. What are the physical states of water at standard temperature and pressure?
Water can exist as a solid (ice), a liquid (water), and a gas (water vapor) under standard conditions.
2. Why does water have a high specific heat capacity?
Water has a high specific heat capacity because it can absorb and store a significant amount of heat energy without a substantial change in temperature. This property helps regulate Earth’s temperature and climate.
3. What is the heat of vaporization, and why is it important for water?
The heat of vaporization is the amount of energy required to change a substance from a liquid to a gas at a constant temperature. Water’s high heat of vaporization is important because it makes sweating an effective cooling mechanism for living organisms.
4. Can you explain the density anomaly of water?
Water’s density decreases as it freezes, causing ice to float on water. This anomaly is vital for aquatic life, as it insulates bodies of water during freezing temperatures.
5. What is the significance of water’s cohesion and adhesion properties?
Water’s cohesion allows its molecules to stick together, while adhesion allows them to adhere to other surfaces. These properties are responsible for capillary action in plants and the formation of the meniscus in containers.
Alcohols Phenols And Ethers Class 12
Alcohols Phenols And Ethers Class 12: Alcohols, phenols, and ethers are fundamental classes of organic compounds that play crucial roles in various chemical, industrial, and biological processes.
These compounds are characterized by the presence of oxygen atoms bonded to carbon atoms, creating a wide range of chemical properties and applications. In this article, we will delve into the structures, nomenclature, properties, and applications of alcohols, phenols, and ethers.

Alcohols Phenols And Ethers
I. Alcohols
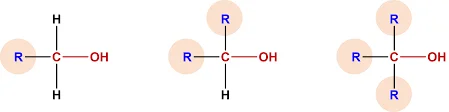
Structure and Nomenclature: Alcohols are organic compounds that contain a hydroxyl (-OH) group attached to a carbon atom. The general formula for alcohols is R-OH, where R represents an alkyl or aryl group. Alcohols are named by replacing the -e at the end of the alkane name with -ol. For example, methane becomes methanol when a hydroxyl group is attached.
Properties:
- Alcohols exhibit a wide range of physical properties depending on the size and structure of the alkyl group. They can be liquids or solids at room temperature.
- Hydrogen bonding between the hydroxyl groups makes alcohols highly soluble in water.
- Alcohols can undergo various chemical reactions, including oxidation, esterification, and dehydration.
Applications:
- Methanol and ethanol are widely used as solvents in industries and laboratories.
- Ethanol is a common alcohol used in alcoholic beverages and as a biofuel.
- Alcohols are crucial intermediates in the synthesis of pharmaceuticals, plastics, and perfumes.
II. Phenols
Structure and Nomenclature: Phenols are organic compounds with a hydroxyl group (-OH) attached directly to an aromatic benzene ring. The general formula for phenols is Ar-OH, where Ar represents an aromatic group. Phenols are typically named by adding the word “phenol” in front of the substituents on the benzene ring.
Properties:
- Phenols are slightly acidic due to the presence of the hydroxyl group, which can release a proton (H+) into solution.
- They have a distinct odor and are often used in perfumes and antiseptic products.
- Many phenolic compounds exhibit antioxidant properties.
Applications:
- Phenol itself is used in the production of resins, plastics, and pharmaceuticals.
- Compounds like cresol and xylenol are used as disinfectants.
- Natural phenols, such as those found in green tea and fruits, are known for their health benefits.

III. Ethers
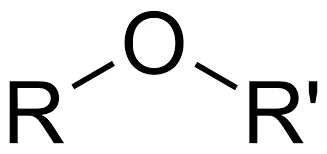
Structure and Nomenclature: Ethers consist of two organic groups (alkyl or aryl) bonded to an oxygen atom, which serves as the bridge between them. The general formula for ethers is R-O-R’, where R and R’ represent alkyl or aryl groups. Ethers are named by listing the two organic groups on either side of the oxygen atom, followed by the word “ether.”
Properties:
- Ethers have relatively low boiling points and are often volatile compounds.
- They are less polar than alcohols and less soluble in water but highly flammable.
- Ethers are relatively stable compounds.
Applications:
- Diethyl ether, historically known as “ether,” was once widely used as a general anesthetic.
- Ethers are used as solvents in laboratories and industries.
- Dimethyl ether (DME) is gaining attention as a clean-burning alternative fuel.
Conclusion
Alcohols, phenols, and ethers are essential organic compounds with diverse structures and properties, making them invaluable in various fields, including chemistry, industry, medicine, and everyday life. Their versatility in chemical reactions and their role as intermediates in the synthesis of various products underscore their importance in the world of organic chemistry. Understanding these compounds is essential for scientists and chemists working in a wide range of applications.
Read More
- Alcohols Phenols And Ethers
- Effect Of Magnet On Current Carying Wire
- Simple Harmonic Motion Formulas
- Dipole Uniform Magnetic Field
- Light Filtering Polaroid Films Polarization
Frequently Asked Questions (FAQs) Alcohols Phenols And Ethers
1. What are alcohols, phenols, and ethers?
Alcohols are organic compounds characterized by the presence of a hydroxyl (-OH) group attached to a carbon atom. Phenols are similar but have the -OH group directly attached to an aromatic benzene ring. Ethers consist of two organic groups bonded to an oxygen atom.
2. How are alcohols named?
Alcohols are typically named by replacing the -e at the end of the alkane name with -ol. For example, methane becomes methanol when a hydroxyl group is attached.
3. Are alcohols soluble in water?
Yes, alcohols are often highly soluble in water due to hydrogen bonding between the hydroxyl groups and water molecules.
4. What is the acidity of phenols?
Phenols are slightly acidic because they can release a proton (H+) into solution due to the presence of the hydroxyl group.
5. What is the main characteristic of ethers?
Ethers are characterized by the presence of an oxygen atom that serves as a bridge between two organic groups.
Difference Between Isothermal And Adiabatic Process
Difference Between Isothermal And Adiabatic Process: Certainly! Here’s a concise summary of the difference between isothermal and adiabatic processes:

Difference Between Isothermal And Adiabatic Process
Isothermal Process:
- Temperature: Temperature remains constant throughout the process.
- Heat Transfer: Heat is exchanged with the surroundings to maintain temperature.
- Efficiency: Isothermal processes are more efficient due to constant temperature.
- Applications: Used in refrigeration and precise chemical reactions.
- Mathematical Representation: Follows the equation PV = constant for ideal gases.

Adiabatic Process:
- Temperature: Temperature can change significantly during the process.
- Heat Transfer: No heat exchange with the surroundings; the process is thermally isolated.
- Efficiency: Adiabatic processes are generally less efficient due to temperature changes.
- Applications: Common in gas compression and expansion, like internal combustion engines.
- Mathematical Representation: Follows the equation PV^γ = constant, where γ is the ratio of specific heats.
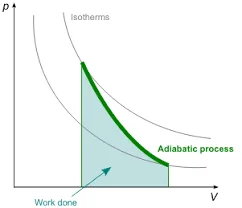
In summary, the key distinction lies in temperature behavior and heat transfer. Isothermal processes maintain constant temperature with heat exchange, while adiabatic processes have temperature changes without heat exchange.
Read More
- Simple Harmonic Motion Examples
- Molecular Mass Of Calcium
- Molecular Weight of Chlorine
- Molecular Weight Of Benzene
- Lumen Meaning In Biology
Frequently Asked Questions (FAQs) Difference Between Isothermal And Adiabatic Process
1. What is an isothermal process?
An isothermal process is one in which the temperature of a system remains constant throughout the process. Heat is continuously added or removed to maintain this constant temperature.
2. What characterizes an adiabatic process?
An adiabatic process is defined by the absence of heat exchange with the surroundings. It occurs in thermally isolated systems where no heat is added to or removed from the system.
3. How does temperature change in an isothermal process?
In an isothermal process, the temperature remains constant, so there is no significant change in temperature.
4. Can you explain how temperature changes in an adiabatic process?
In an adiabatic process, temperature can change significantly. When work is done on or by the system, it can lead to temperature increases (compression) or decreases (expansion) without any heat exchange with the surroundings.
5. Which process is more efficient, isothermal or adiabatic?
Isothermal processes are generally more efficient because they maintain a constant temperature, allowing for maximum energy transfer. Adiabatic processes can be less efficient due to temperature changes.
Difference Between Fuse And Circuit Breaker
Difference Between Fuse And Circuit Breaker: Fuses and circuit breakers are both electrical safety devices designed to protect electrical circuits from overcurrents and short circuits, but they differ in how they operate and their resetability. Here’s a comparison of the key differences between fuses and circuit breakers:
Difference Between Fuse And Circuit Breaker
1. Operation:
- Fuse: A fuse is a simple, one-time-use device that consists of a thin wire or metal strip that melts when exposed to excessive current. When the current exceeds a certain threshold, the fuse wire melts, breaking the circuit and disconnecting the power.
- Circuit Breaker: Circuit breakers are reusable devices that can be manually reset after they trip. They use an electromechanical mechanism to detect overcurrent or short-circuit conditions. When a fault occurs, the circuit breaker trips, opening the circuit to interrupt the current flow.
2. Resetability:
- Fuse: Fuses are not resettable. Once a fuse melts and interrupts the circuit, it needs to be replaced with a new fuse of the same rating.
- Circuit Breaker: Circuit breakers are resettable. After they trip due to a fault, they can be reset by flipping the breaker’s switch back to the “on” position, restoring power to the circuit.
3. Cost:
- Fuse: Fuses are generally less expensive than circuit breakers, but the cost adds up over time since they need to be replaced each time they blow.
- Circuit Breaker: Circuit breakers are more expensive upfront but offer long-term cost savings because they can be reset and reused.
4. Response Time:
- Fuse: Fuses typically have a faster response time than circuit breakers since they react almost instantly to overcurrent conditions.
- Circuit Breaker: Circuit breakers may have a slightly slower response time because of the mechanical components involved, but they are still effective in protecting against overcurrents.
5. Sensitivity:
- Fuse: Fuses are generally less sensitive to minor overcurrents and may not trip as quickly as circuit breakers in response to small faults.
- Circuit Breaker: Circuit breakers can be adjusted to different levels of sensitivity, allowing for better protection against a wider range of faults.
6. Lifespan:
- Fuse: Fuses have a limited lifespan and need replacement each time they blow.
- Circuit Breaker: Circuit breakers have a longer lifespan and can be used repeatedly as long as they are not damaged.
In summary, fuses and circuit breakers both serve the essential purpose of protecting electrical circuits, but they differ in their operation, resetability, cost, response time, sensitivity, and lifespan. The choice between them depends on the specific requirements of the electrical system and the desired level of protection.


Read More
- Difference Between Electromagnet And Permanent Magnet
- Blind Visually Impaired Optical Low Vision Aids
- Bar Magnet As An Equivalent Solenoid
- Equivalent Weight of KMnO4
- Molecular Mass of Na
Frequently Asked Questions (FAQs) Difference Between Fuse And Circuit Breaker
1. What is a fuse, and how does it work?
A fuse is an electrical safety device that consists of a thin wire or metal strip. It works by melting when exposed to excessive current, breaking the circuit and disconnecting the power.
2. What is a circuit breaker, and how does it work?
A circuit breaker is an electrical safety device that uses an electromechanical mechanism to detect overcurrent or short-circuit conditions. It trips by opening the circuit to interrupt the current flow.
3. Can fuses be reset after they blow?
No, fuses are one-time-use devices and cannot be reset. They need to be replaced with a new fuse of the same rating.
4. Can circuit breakers be reset after they trip?
Yes, circuit breakers are resettable. After they trip due to a fault, they can be manually reset by flipping the breaker’s switch back to the “on” position.
5. Which is more cost-effective, fuses, or circuit breakers?
Fuses are generally less expensive upfront than circuit breakers. However, circuit breakers offer long-term cost savings because they can be reset and reused, whereas fuses need replacement.
Difference Between Electromagnet And Permanent Magnet
Difference Between Electromagnet And Permanent Magnet: Electromagnets and permanent magnets are two distinct types of magnets with significant differences in their properties, generation, and applications. Here’s a breakdown of the key differences between them:

Difference Between Electromagnet And Permanent Magnet
1. Generation:
- Electromagnets: are magnetism on demand, formed by directing an electric current through a coiled wire. The presence of current generates a magnetic field, and once the current ceases, the magnetism promptly dissipates.
- Permanent Magnet: Permanent magnets are naturally occurring or man-made materials that retain their magnetism without the need for an external electric current. They have a persistent magnetic field.
2. Magnetic Strength:
- Electromagnet: The strength of an electromagnet can be easily adjusted by changing the amount of current passing through the coil. Strong electromagnets can be created for specific applications.
- Permanent Magnet: The strength of a permanent magnet is fixed and cannot be easily altered without demagnetizing or physically altering the magnet.
3. Durability:
- Electromagnet: Electromagnets are not as durable as permanent magnets since their magnetic properties depend on the presence of an electric current. When the current is interrupted, the magnetism disappears.
- Permanent Magnet: Permanent magnets are more durable and can maintain their magnetism for a long time under normal conditions.
4. Applications:
- Electromagnet: Electromagnets are commonly used in applications where the magnetic field needs to be controlled or switched on and off, such as in electric motors, solenoids, MRI machines, and magnetic locks.
- Permanent Magnet: Permanent magnets are used in various applications, including refrigerator magnets, magnetic compasses, speakers, hard drives, and generators.
5. Size and Shape:
- Electromagnets: Electromagnets offer flexibility in design, allowing for customization in terms of size and shape to suit specific application needs.
- Permanent Magnet: On the other hand, permanent magnets also come in various sizes and shapes, but their magnetic characteristics are inherently defined by the materials they are composed of.
6. Maintenance:
- Electromagnets: often necessitate routine maintenance to safeguard the integrity of the coil and its connections. Furthermore, their magnetism can diminish if the coil sustains damage or if there is a failure in the power source.
- Permanent Magnet: Permanent magnets generally require little to no maintenance as long as they are not subjected to extreme conditions that could demagnetize them.
In summary, the primary difference between electromagnets and permanent magnets lies in their generation, magnetic strength control, durability, and applications. Electromagnets derive their magnetism from an electric current, making them temporary magnets. In contrast, permanent magnets possess an inherent magnetic field and do not depend on external power sources to sustain their magnetism.
Read More
- Blind Visually Impaired Optical Low Vision Aids
- Bar Magnet As An Equivalent Solenoid
- Equivalent Weight of KMnO4
- Molecular Mass of Na
- Chemical Properties of Acid
Frequently Asked Questions (FAQs) On Difference Between Electromagnet And Permanent Magnet
1. What is an electromagnet?
An electromagnet is a temporary magnet created by passing an electric current through a coil of wire.
2. What is a permanent magnet?
A permanent magnet is a magnet that retains its magnetic properties without the need for an external electric current.
3. How are electromagnets and permanent magnets generated differently?
Electromagnets are generated by passing an electric current through a coil of wire, while permanent magnets are naturally occurring or man-made materials with intrinsic magnetic properties.
4. Can you control the strength of an electromagnet?
Yes, the strength of an electromagnet can be easily adjusted by changing the amount of current passing through the coil.
5. Can you control the strength of a permanent magnet?
No, the strength of a permanent magnet is fixed and cannot be easily altered without demagnetizing or physically altering the magnet.
Blind Visually Impaired Optical Low Vision Aids
Blind Visually Impaired Optical Low Vision Aids: Visual impairment, whether from birth or acquired later in life, presents unique challenges for individuals in their daily lives.
Tasks that many of us take for granted, such as reading a book, recognizing faces, or safely navigating the environment, can become significantly more difficult for those with visual impairments. However, advancements in technology and innovative assistive devices have greatly improved the quality of life for the blind and visually impaired. Among these aids, optical low vision aids play a crucial role in enhancing independence and accessibility.
This article delves into the world of optical low vision aids, explaining what they are, how they work, and their various types and applications. We’ll also explore how these aids are changing the lives of individuals with visual impairments and providing new opportunities for education, employment, and overall well-being.

Blind Visually Impaired Optical Low Vision Aids
Blind Visually Impaired Optical Low Vision Aids
Understanding Optical Low Vision Aids
Optical low vision aids are precisely engineered devices created to empower individuals who are blind or have visual impairments, enabling them to make the most of their remaining vision.. They use optical technology to magnify and enhance images, making them more accessible to those with visual impairments. These aids are indispensable tools that bridge the gap between standard eyeglasses or contact lenses and more complex vision restoration technologies like surgery or implants.
Types of Optical Low Vision Aids
There is a wide range of optical low vision aids available, each catering to specific visual needs and preferences. Here are some of the most common types:
- Magnifiers: Magnifiers use lenses to enlarge and enhance the appearance of objects. They come in various forms, such as handheld magnifiers, stand magnifiers, and illuminated magnifiers. Some are portable for on-the-go use, while others are designed for specific tasks like reading or viewing distant objects.
- Telescopes: Telescopic aids are designed to help individuals see distant objects more clearly. They are often used for activities like watching sports events, enjoying scenic views, or identifying objects at a distance.
- Electronic Magnification Devices: These devices, often referred to as video magnifiers or closed-circuit television (CCTV) systems, use cameras and displays to magnify printed materials or objects. Users can adjust the magnification level, contrast, and color settings to suit their needs.
- Wearable Aids: Some optical low vision aids are wearable and utilize cameras and displays to enhance the user’s vision. These devices can be particularly helpful for individuals with conditions like retinitis pigmentosa.
- Reading Glasses with Built-in Magnification: These are traditional-looking eyeglasses with magnifying lenses built into them, making it easier for individuals to read small print or engage in close-up tasks.
How Optical Low Vision Aids Work
- The basic principle behind optical low vision aids is the magnification of objects to make them more visible. Here’s a simplified explanation of how some of these aids work:
- Magnifiers: A magnifier uses a convex lens to bend and enlarge light, making objects appear larger and closer to the user’s eyes. The user positions the magnifier between their eyes and the object they want to see, adjusting the distance for optimal focus.
- Telescopes: Telescopic aids use a combination of lenses to bring distant objects into focus. Users can adjust the eyepiece or settings to achieve the desired magnification.
- Electronic Magnification Devices: These devices capture an image using a camera, display it on a screen, and allow the user to zoom in, change contrast, and apply other settings to enhance visibility.
- Wearable Aids: Wearable devices, such as electronic glasses, employ cameras to capture real-time video and project it onto screens positioned in front of the user’s eyes. These screens are customizable, allowing users to adjust settings for magnification and contrast to suit their specific visual preferences.
Applications and Impact
Optical low vision aids have a profound impact on the lives of individuals with visual impairments:
- Education: These aids enable students with visual impairments to access textbooks, study materials, and classroom presentations more easily. They promote independent learning and academic success.
- Employment: Many individuals with visual impairments use optical low vision aids to perform tasks at work, enhancing their job performance and career opportunities.
- Daily Living: Optical low vision aids assist with everyday activities such as reading, cooking, recognizing faces, and safely navigating unfamiliar environments.
- Leisure and Hobbies: These aids empower individuals to pursue hobbies like reading, watching TV, knitting, or enjoying outdoor activities with greater ease and enjoyment.
- Social Engagement: Enhanced vision fosters better social interaction, allowing individuals to recognize friends and family, read expressions, and engage in social activities.
- Healthcare: Optical aids help individuals manage their health by reading medication labels, monitoring their vital signs, and accessing healthcare information.
Consulting a Low Vision Specialist
While certain optical low vision aids are available for purchase without a prescription, it is highly recommended that individuals with visual impairments seek consultation with an eye care professional or a low vision specialist. These specialized professionals can offer tailored advice and recommend the most appropriate aids, including prescribing specific devices that align with the individual’s unique needs and requirements.
Conclusion
Optical low vision aids have revolutionized the lives of individuals who are blind or visually impaired. These devices enhance independence, accessibility, and overall quality of life by making the most of the user’s remaining vision. As technology continues to advance, the future looks brighter for individuals with visual impairments, offering them new opportunities. Experiences previously thought impossible.
Read More
- Bar Magnet As An Equivalent Solenoid
- Equivalent Weight of KMnO4
- Molecular Mass of Na
- Chemical Properties of Acid
- Molar Mass of Benzene
Frequently Asked Questions (FAQs) Blind Visually Impaired Optical Low Vision Aids
What are optical low vision aids for the blind and visually impaired?
Optical low vision aids are devices designed to assist individuals who are blind or visually impaired in enhancing their remaining vision. These aids use optical technology to magnify and enhance images, making them more accessible to those with visual impairments.
Who can benefit from optical low vision aids?
Optical low vision aids can benefit individuals with a range of visual impairments, including those with conditions like macular degeneration, glaucoma, diabetic retinopathy, and other causes of low vision. These aids are often use when standard glasses or contact lenses are no longer effective.
What types of optical low vision aids are available?
There are several types of optical low vision aids, including magnifiers, telescopes, electronic magnification devices, and reading glasses with built-in magnification. The choice of aid depends on an individual’s specific visual needs and preferences.
How do magnifiers work for low vision users?
Magnifiers employ lenses to enlarge and improve the visibility of objects. They are available in diverse forms, including handheld magnifiers, stand magnifiers, and illuminated magnifiers. Some models are specifically engineered with portability in mind, making them well-suited for use while on the move, while others are carefully crafted to excel in specialized tasks such as reading or observing distant objects.
What are electronic magnification devices?
Electronic magnification devices, often referred to as video magnifiers or closed-circuit television (CCTV) systems. Use cameras and displays to magnify printed materials or objects. Users can adjust the magnification level, contrast, and color settings to suit their needs.
Bar Magnet As An Equivalent Solenoid
Bar Magnet As An Equivalent Solenoid: Magnetism is a fundamental force of nature that has intrigued scientists and researchers for centuries.
It plays a vital role in numerous everyday applications, from the magnetic locks on your doors to the functionality of your computer’s hard drive.
To understand magnetism better, scientists have developed various models and concepts, including the idea of magnetic equivalence between a bar magnet and a solenoid.
Bar Magnet As An Equivalent Solenoid
1. Bar Magnets: A Primer
Before we delve into the magnetic equivalence, it’s essential to understand the basics of bar magnets, including their magnetic properties, field lines, and dipole moment.

Magnetic Properties of Bar Magnets
A bar magnet is a permanent magnet with two distinct poles: a north pole (N) and a south pole (S). These poles exhibit attractive or repulsive forces when brought near other magnets or magnetic materials. The magnetic strength of a bar magnet depends on various factors, including its material composition and size.
One of the key properties of bar magnets is their ability to align themselves in a north-south direction due to Earth’s magnetic field. This alignment is a manifestation of their inherent magnetic properties.
Magnetic Field Lines
To visualize the magnetic field around a bar magnet, scientists use magnetic field lines. These lines provide a graphical representation of the magnetic field’s direction and strength. The magnetic field lines of a bar magnet emerge from the north pole and enter the south pole, forming closed loops.
These field lines provide a clear depiction of how the magnetic field varies in strength and direction in the vicinity of the magnet. The field lines are closer together near the poles, indicating a stronger magnetic field, and they spread out as they move farther from the magnet.
Magnetic Poles and Dipole Moment
A bar magnet’s magnetic behavior can be explained using the concept of a magnetic dipole. A magnetic dipole consists of two poles of opposite polarity separated by a small distance. In the case of a bar magnet, the two magnetic poles (north and south) create a magnetic dipole.
The strength of the magnetic dipole moment () of a bar magnet is determined by the product of the magnetic pole strength () and the distance between the poles ():
m = mp ⋅ d
The direction of the dipole moment points from the south pole to the north pole. Understanding the concept of a magnetic dipole is crucial for comprehending the magnetic behavior of bar magnets.
2. Solenoids: The Magnetic Coils
Solenoids are another essential component of our exploration of magnetic equivalence. These are coils of wire that, when a current flows through them, generate a magnetic field. Let’s dive into the structure of solenoids, their magnetic fields, and the role of current in creating magnetic effects.
Structure and Components of a Solenoid
A solenoid is essentially a long, cylindrical coil of wire with multiple turns. It can be thought of as a tightly wound wire helix. When an electric current flows through the wire, it creates a magnetic field along the axis of the solenoid. The strength of the magnetic field depends on several factors, including the number of turns in the coil, the current passing through it, and the nature of the core material (if present).
Solenoids are commonly used in various electromechanical devices, such as electromagnetic locks, relays, and even in the starters of internal combustion engines.
Magnetic Field Inside a Solenoid
The magnetic field inside a solenoid is uniform, meaning it has a consistent strength and direction throughout the interior of the coil. This magnetic field is similar to the field generated by a bar magnet. When current flows through the solenoid, it induces a magnetic field that aligns with the direction of the coil’s axis.
The strength of the magnetic field inside a solenoid () is determined by several factors, including the number of turns in the coil (), the current passing through it (), and a physical constant known as permeability of free space ():
B=μ0 ⋅ N ⋅ I/L
Where:
- is the magnetic field strength inside the solenoid.
- is the permeability of free space, a constant approximately equal to 4×10−7T·m/A.
- is the number of turns of wire in the solenoid.
- is the current flowing through the solenoid.
- is the length of the solenoid.
This equation demonstrates how the magnetic field strength can be controlled and increased by adjusting the current or the number of turns in the solenoid.
The Role of Current in Creating a Magnetic Field
The key factor responsible for generating a magnetic field in a solenoid is the flow of electric current through the wire coil. As the current passes through each turn of the coil, it creates a small magnetic field. The cumulative effect of these individual magnetic fields from each turn results in a net magnetic field that is stronger and more directed along the axis of the solenoid.
The direction of the magnetic field inside the solenoid is determined by the direction of the current flow. The right-hand rule, which is a common mnemonic in physics, helps determine the direction of the magnetic field relative to the current direction. According to the right-hand rule, if you grasp the solenoid with your right hand so that your thumb points in the direction of the current flow, your fingers will curl around the solenoid in the direction of the magnetic field lines.
3. The Magnetic Equivalence
Now that we have explored the fundamental properties of bar magnets and solenoids, we can begin to unravel the concept of magnetic equivalence and how it relates these two magnetic entities.
Ampere’s Circuital Law
Ampere’s Circuital Law is a fundamental principle in electromagnetism that establishes a relationship between the magnetic field produced by a current-carrying conductor and the geometry of that conductor. The law, formulated by André-Marie Ampère in the early 19th century, provides a basis for understanding the magnetic field generated by a solenoid.
Ampere’s Circuital Law states that the line integral of the magnetic field () around a closed path (a closed loop) is equal to the product of the current () passing through the loop and the constant permeability of free space ():
∮B ⋅ dl = μ0 ⋅ Ienc
Where:
- represents the line integral of the magnetic field around the closed path.
- is the permeability of free space.
- is the total enclosed current passing through the loop.
Ampere’s Circuital Law provides a mathematical framework for understanding the magnetic field around a closed loop created by a current-carrying conductor. This law is particularly applicable to solenoids.
Magnetic Moments in Bar Magnets and Solenoids
To establish the magnetic equivalence between a bar magnet and a solenoid, we need to consider the magnetic moments of both entities. The magnetic moment () of an object is a vector quantity that represents the strength and orientation of its magnetic poles.
In a bar magnet, the magnetic moment () is determined by the product of the magnetic pole strength () and the distance between the poles ():
mbar magnet = mp ⋅ d
In a solenoid, the magnetic moment () is determined by the product of the magnetic field strength inside the solenoid () and the cross-sectional area () of the solenoid:
msolenoid = B ⋅ A
Now, let’s examine how Ampere’s Circuital Law and the concept of equivalent solenoid bridge the gap between these two magnetic moments.
The Concept of Equivalent Solenoid
The concept of an equivalent solenoid is a powerful idea in electromagnetism. It states that a long, tightly wound solenoid can be used to approximate the magnetic behavior of a bar magnet. In other words, if you have a sufficiently long solenoid with a large number of turns and a current passing through it, it can create a magnetic field similar to that of a bar magnet.
This magnetic equivalence is rooted in Ampere’s Circuital Law. When you pass a current through a solenoid, it generates a magnetic field that is proportional to the current and the number of turns in the solenoid, as described earlier. This magnetic field can be strong and uniform if the solenoid is long enough and has enough turns.
Now, consider a closed loop encircling the solenoid. According to Ampere’s Circuital Law, the line integral of the magnetic field () around this loop is equal to the product of the current () passing through the loop and the permeability of free space ():
∮B ⋅ dl = μ0 ⋅ Ienc
In the case of a solenoid, the magnetic field inside the solenoid is nearly uniform, and it points along the axis of the solenoid. This means that the line integral of the magnetic field around the loop is simply the product of the magnetic field strength () and the circumference of the loop (2), where is the radius of the loop:
∮B ⋅ dl = B ⋅ 2πr
Now, consider the right-hand side of Ampere’s Circuital Law, which is the product of the current passing through the loop () and the permeability of free space (0). If we choose the right parameters for our solenoid and loop, we can make equal to the product of the magnetic pole strength () of a bar magnet and the number of turns in the solenoid ():
Ienc = mp ⋅ N
This choice is crucial because it allows us to express Ampere’s Circuital Law in a way that relates the magnetic field of the solenoid () to the magnetic pole strength () of the bar magnet:
B ⋅ 2πr = μ0 ⋅ mp ⋅ N
Now, let’s rearrange the equation:
B = μ0 ⋅ mp ⋅ N/2πr
The term represents the number of turns per unit length of the solenoid (). Therefore, we can rewrite the equation as:
B = μ0 ⋅ mp ⋅ n
This equation reveals that the magnetic field () inside the solenoid is directly proportional to the magnetic pole strength () of the bar magnet and the number of turns per unit length () of the solenoid.
Now, consider the concept of magnetic moment. We know that the magnetic moment () of the solenoid is given by:
msolenoid = B ⋅ A
In this case, represents the cross-sectional area of the solenoid, and is the magnetic field strength inside the solenoid. Substituting the expression for from our previous equation, we get:
msolenoid = (u0 ⋅ mp . n) ⋅ A
This equation relates the magnetic moment of the solenoid () to the magnetic pole strength of the bar magnet (), the number of turns per unit length of the solenoid (), and the cross-sectional area of the solenoid ().
Now, consider a bar magnet with its magnetic moment (bar magnet). The magnetic equivalence between the solenoid and the bar magnet suggests that magnet is equivalent to :
bar magnet = solenoid
Substituting our expression for into the equation:
mp . d = (u0 . mp . n) . A
Here, represents the distance between the magnetic poles of the bar magnet.
Now, let’s simplify the equation:
d = μ0 ⋅ n ⋅ A/1
This equation reveals an important result: The distance between the magnetic poles of the bar magnet () is equal to a certain combination of constants and variables, including the permeability of free space (), the number of turns per unit length of the solenoid (), and the cross-sectional area of the solenoid ().
What this result implies is that the magnetic behavior of the bar magnet, characterized by its magnetic moment () and the distance between its poles (), can be approximated and related to the parameters of the solenoid (, , and ).
This establishes the concept of magnetic equivalence between a bar magnet and a solenoid. A properly designed and configured solenoid can mimic the magnetic behavior of a bar magnet, and this equivalence is grounded in the principles of Ampere’s Circuital Law and magnetic moments.
4. Applications of Magnetic Equivalence
The magnetic equivalence between a bar magnet and a solenoid has a wide range of practical applications in science and engineering. Let’s explore some of these applications and how this concept is put to use in various fields.
Electromagnetic Devices
One of the most common applications of magnetic equivalence is in the design of electromagnetic devices. These devices use the principles of electromagnetism to perform various tasks, such as generating mechanical motion or producing magnetic fields for specific purposes.
In numerous electromagnetic devices, solenoids play a pivotal role in generating magnetic fields capable of attracting or repelling magnetic materials. Engineers can precisely adjust the device’s behavior by regulating the current flowing through the solenoid, thereby controlling the magnetic field’s strength.
As an illustration, electromagnetic door locks rely on a solenoid to generate a potent magnetic field that effectively secures a door when the lock is activated. When the current is deactivated, the magnetic field dissipates, permitting the door to open. This same principle finds application in various automotive systems, including solenoid-operated valves within fuel injection systems.
Magnetic Sensors
Magnetic sensors, such as Hall effect sensors, rely on the detection of magnetic fields to measure various physical quantities. These sensors are commonly used in electronic devices and automotive applications.
In some cases, to provide a stable and known magnetic field for calibration and testing purposes, an equivalent solenoid may be employed. By controlling the current through the solenoid, engineers can precisely control the strength and orientation of the magnetic field, ensuring the accuracy of magnetic sensor measurements.
MRI Scanners
Magnetic Resonance Imaging (MRI) is a medical imaging technique that relies on strong magnetic fields and radio waves to generate detailed images of the body’s internal structures. In an MRI scanner, powerful superconducting solenoids create the magnetic fields necessary for imaging.
The concept of magnetic equivalence between a solenoid and a bar magnet is essential in the design and operation of MRI machines. These solenoids must produce highly uniform and stable magnetic fields to ensure the quality and accuracy of MRI images.
Magnetic Resonance Imaging (MRI)
Magnetic Resonance Imaging (MRI) is a non-invasive medical imaging technique that provides detailed images of the body’s internal structures. MRI scanners use powerful magnetic fields and radio waves to create these images, and they rely on the principles of magnetic equivalence for their operation.
Inside an MRI scanner, superconducting solenoids generate the strong and highly uniform magnetic fields required for imaging. These solenoids are designed to produce magnetic fields that are equivalent to those of ideal bar magnets. The magnetic equivalence ensures that the MRI scanner’s magnetic field is consistent and precise, leading to high-quality images with excellent contrast and resolution.
The concept of magnetic equivalence is integral to the design and calibration of MRI machines, allowing for the accurate and reliable imaging of various medical conditions.
5. Experimental Verification
The concept of magnetic equivalence between a bar magnet and a solenoid can be experimentally verified using simple laboratory setups and equipment. Experimental verification is essential to confirm that the magnetic field produced by a solenoid indeed mimics the behavior of a bar magnet.
Comparing Magnetic Fields
An effective approach to experimentally validating magnetic equivalence is to compare the magnetic fields generated by both a bar magnet and a solenoid. This comparison can be accomplished by employing a magnetic field sensor or a magnetic compass.
- Bar Magnet Setup: Start by measuring the magnetic field strength at different points around a bar magnet using a magnetic field sensor or a magnetic compass. Record the field strengths and directions at various locations near the magnet.
- Solenoid Setup: Next, create a solenoid with a known number of turns per unit length () and a known cross-sectional area (). Pass a current through the solenoid to generate a magnetic field. Measure the magnetic field strength at the same locations where you measured the bar magnet’s field strength. Ensure that the
Read More
- Equivalent Weight of KMnO4
- Molecular Mass of Na
- Chemical Properties of Acid
- Molar Mass of Benzene
- Molar Mass of Calcium
Frequently Asked Questions (FAQs) Bar Magnet As An Equivalent Solenoid
What is the concept of a bar magnet being equivalent to a solenoid?
The concept of magnetic equivalence suggests that a properly designed and configured solenoid can mimic the magnetic behavior of a bar magnet. This means that they produce similar magnetic fields.
What is a magnetic dipole?
A magnetic dipole consists of two magnetic poles of opposite polarity separated by a small distance. In the context of a bar magnet, the north and south poles create a magnetic dipole.
How can the magnetic equivalence between a bar magnet and a solenoid be experimentally verified?
To verify magnetic equivalence, you can compare the magnetic fields generated by a bar magnet and a solenoid using a magnetic field sensor or a magnetic compass. This experimental setup helps confirm the similarity of their magnetic behaviors.
What are some practical applications of the concept of magnetic equivalence?
The concept of magnetic equivalence between a bar magnet and a solenoid finds applications in various fields, including the development of electromagnetic devices, magnetic sensors, and the operation of Magnetic Resonance Imaging (MRI) scanners within the medical field.
Why is understanding magnetic equivalence important in engineering and physics?
Understanding magnetic equivalence is crucial for designing and using devices that rely on magnetic fields. It allows engineers and physicists to predict and control magnetic behaviors, leading to the development of innovative technologies.
Molecular Weight Of K2Cr2O7
Molecular Weight Of K2Cr2O7: Potassium dichromate, with the chemical formula K2Cr2O7, is a chemical compound widely used in various industries and laboratories for its oxidizing properties.
Understanding the molecular weight of potassium dichromate is essential in chemistry, particularly in analytical and stoichiometric calculations. In this article, we will delve into the mole weight of K2Cr2O7, its significance, and its applications in different fields.

Molecular Weight Of K2Cr2O7
Exploring the Atomic Structure of Potassium Dichromate (K2Cr2O7)
Before we calculate the mole weight of potassium dichromate, it’s crucial to examine its atomic composition:
- Potassium (K): Potassium has an atomic mass of approximately 39.10 atomic mass units (amu).
- Chromium (Cr): Chromium has an atomic mass of approximately 51.99 amu.
- Oxygen (O): Oxygen has an atomic mass of approximately 16.00 amu.
Potassium dichromate (K2Cr2O7) consists of two potassium atoms (K), two chromium atoms (Cr), and seven oxygen atoms (O).
Calculating the Molecular Weight of K2Cr2O7
To calculate the mole weight of potassium dichromate (K2Cr2O7), we sum the atomic masses of its constituent atoms according to the chemical formula:
Molecular Weight of K2Cr2O7 = (2 × Atomic Mass of K) + (2 × Atomic Mass of Cr) + (7 × Atomic Mass of O)
Molecular Weight of K2Cr2O7 = (2 × 39.10 amu) + (2 × 51.99 amu) + (7 × 16.00 amu)
The Molecular Weight of K2Cr2O7 = 78.20 amu + 103.98 amu + 112.00 amu
Molecular Weight of K2Cr2O7 ≈ 294.18 amu
Therefore, the molecular weight of potassium dichromate (K2Cr2O7) is approximately 294.18 atomic mass units (amu) or 294.18 grams per mole (g/mol).
Significance of Molecular Weight in Potassium Dichromate
The molecular weight of potassium dichromate is significant for various reasons:
- Analytical Chemistry: Potassium dichromate is widely used as a primary standard in titrations, particularly for the determination of reducing agents. Its accurate molecular weight is vital for precise volumetric analysis.
- Environmental Analysis: It finds application in laboratories dedicated to environmental testing, where it is instrumental in analyzing diverse water and soil samples. Its primary role is in accurately assessing the concentrations of organic compounds and pollutants.
- Safety Considerations: Potassium dichromate is a hazardous chemical with various industrial applications, including as a corrosion inhibitor and in the manufacturing of pigments. Knowledge of its molecular weight aids in proper handling and safety protocols.
- Educational and Research Purposes: In educational settings and research laboratories, potassium dichromate is used for experiments and studies related to redox reactions and stoichiometry.
Conclusion
The molecular weight of potassium dichromate (K2Cr2O7), approximately 294.18 amu or 294.18 g/mol, is a fundamental property that underlies its applications in chemistry, analytical science, environmental testing, and industry. Whether it’s ensuring the accuracy of analytical results, adhering to safety guidelines, or conducting research, understanding the molecular weight of potassium dichromate is crucial for achieving reliable and meaningful outcomes in various scientific and practical endeavors.
Read More
- Molecular Weight Of Phosphorus
- Molecular Weight Of SO2
- Molecular Weight of Na2CO3
- Molecular Mass Of Sodium
- Molecular Mass of Sucrose
Frequently Asked Questions (FAQs) Molecular Weight Of K2Cr2O7
What is the molecular weight of potassium dichromate (K2Cr2O7)?
The mole weight of potassium dichromate (K2Cr2O7) is roughly 294.18 atomic mass units (amu) or 294.18 grams per mole (g/mol). This determination is made by summing the atomic masses of its constituent atoms, as specified by its chemical formula.
What is potassium dichromate used for?
Potassium dichromate serves diverse purposes, functioning as an oxidizing agent in chemical reactions, a primary standard in analytical chemistry, a corrosion inhibitor, and a constituent in pigment manufacturing. Additionally, it plays a pivotal role in environmental testing.
Why is it important to know the molecular weight of K2Cr2O7?
Understanding the molecular weight of potassium dichromate is crucial for precise analytical chemistry, accurate dosing in experiments, and adhering to safety guidelines. It ensures the correct proportions of reactants and products in chemical reactions.
Is potassium dichromate hazardous?
Indeed, potassium dichromate is classified as a hazardous chemical due to its toxic, carcinogenic properties. Its potential to induce severe skin and respiratory irritation. When working with it, it is imperative to adhere to strict safety protocols, which include the use of personal protective equipment.
What are the environmental applications of potassium dichromate?
Potassium dichromate finds application in environmental testing laboratories for the examination of water and soil samples, where its significance lies in accurately assessing the concentrations of organic compounds and pollutants.
Molecular Weight Of Phosphorus
Molecular Weight of Phosphorus: Phosphorus, symbolized by “P” in the periodic table, is a fundamental element present in all living organisms and holds a central position in numerous biochemical processes.
Understanding the mole weight of phosphorus is fundamental in chemistry, biology, and environmental science, as it provides insights into the behavior of this element in different contexts. In this article, we will explore the mole weight of phosphorus, its significance, and its diverse applications.

Molecular Weight Of Phosphorus
Exploring the Atomic Structure of Phosphorus
Before delving into the mole weight of phosphorus, it’s essential to examine its atomic structure:
- Atomic Number: Phosphorus is defined by its atomic number, which is 15. This indicates that a phosphorus atom harbors 15 protons within its nucleus.
- Electron Configuration: The electron configuration of phosphorus is 2-8-5, meaning it has two electrons in its first energy level, eight in its second energy level, and five in its third energy level.
- Isotopes: Phosphorus has several isotopes, with phosphorus-31 (P-31) being the most common and stable isotope. P-31 has 16 neutrons in addition to its 15 protons.
Calculating the Molecular Weight of Phosphorus (P)
To calculate the mole weight of phosphorus (P), we consider the atomic mass of its most abundant and stable isotope, phosphorus-31 (P-31):
- The atomic mass of phosphorus-31 is approximately 30.97 atomic mass units (amu) or 30.97 grams per mole (g/mol).
- Therefore, the mole weight of phosphorus (P) is approximately 30.97 g/mol or 30.97 amu.
Significance of Molecular Weight in Phosphorus
The mole weight of phosphorus holds significant importance in various fields and applications:
- Biochemistry: Phosphorus is a crucial component of DNA, RNA, and ATP (adenosine triphosphate), which are essential molecules in living organisms. Understanding its molecular weight aids in studying these biomolecules and their functions.
- Agriculture: Phosphorus stands as an indispensable nutrient crucial for fostering plant growth and is widely employed in fertilizers. Understanding its molecular weight holds significant importance in the formulation of fertilizers and agricultural strategies.
- Chemical Reactions: Phosphorus participates in various chemical reactions, such as the synthesis of phosphoric acid (used in the production of soft drinks and detergents). Its molecular weight is essential for stoichiometry in chemical equations.
- Environmental Science: Phosphorus is a concern in environmental science due to its role in water pollution (e.g., eutrophication). Understanding its molecular weight is crucial for monitoring and mitigating its impact on aquatic ecosystems.
Conclusion
The molecular weight of phosphorus, approximately 30.97 g/mol or 30.97 amu, is a fundamental property that underpins its significance in the fields of chemistry, biology, and environmental science. Whether it’s its role in DNA, its use as a fertilizer, its participation in chemical reactions, or its impact on aquatic environments, understanding the molecular weight of phosphorus is essential for unraveling its multifaceted behavior and applications. This versatile element continues to be a cornerstone of scientific and practical advancements in various disciplines.
Read More
- Molecular Weight Of SO2
- Molecular Weight of Na2CO3
- Molecular Mass Of Sodium
- Molecular Mass of Sucrose
- Molecular Mass of Chlorine
Frequently Asked Questions (FAQs) Molecular Weight Of Phosphorus
What is the molecular weight of phosphorus?
The molecular weight of phosphorus (P) is roughly 30.97 grams per mole (g/mol) or 30.97 atomic mass units (amu). This determination is based on the atomic mass of its most prevalent and stable isotope, phosphorus-31 (P-31).
Why is it important to know the molecular weight of phosphorus?
Understanding the molecular weight of phosphorus is crucial in various scientific fields. It aids in stoichiometry in chemical reactions, is essential in the study of biochemical processes, and is significant in agriculture for fertilizer formulation.
What are the key roles of phosphorus in biological systems?
Phosphorus plays a pivotal role in biological systems, serving as an essential component within DNA, RNA, and ATP (adenosine triphosphate). These molecules are central to genetic information storage and energy transfer processes. Additionally, phosphorus is of critical importance in maintaining the structure of cell membranes.
How is phosphorus utilized in agriculture?
Phosphorus is an essential nutrient for plant growth. It is commonly used in the form of phosphorus-containing fertilizers to enhance crop yield and quality. Phosphorus promotes root development, flowering, and fruiting in plants.
What is the significance of phosphorus in chemical reactions?
Phosphorus is actively involved in numerous chemical reactions, one of which is the production of phosphoric acid. This acid finds application in the manufacturing of soft drinks and detergents. The molecular weight of phosphorus is of paramount importance in stoichiometric calculations, ensuring the precise ratios of reactants and products in chemical processes.
